Fortune's Formula (35 page)
Authors: William Poundstone
Tags: #Business & Economics, #Investments & Securities, #General, #Stocks, #Games, #Gambling, #History, #United States, #20th Century

A
S APPEALING AS
the Frankenstein image may be, it is hard to draw a practical moral from it. Portfolio managers are no more going to abandon computer models than mobile phones. Software is just a tool for implementing policies that humans have decided are reasonable.
Probably the best single-word explanation for what went wrong at LTCM is
overbetting
. Overbetting (unlike leverage, fat tails, or even a certain amount of healthy self-esteem a.k.a. hubris) is
always
bad.
Overbetting is a concept from gambling, not standard economic theory. Its role in the LTCM debacle was hard to ignore, with two Nobel laureates crawling out of the wreckage. Since 1998 the academic world has studied LTCM’s collapse exhaustively. After years of relative neglect, arbitrage and hedge funds have become objects of serious study. Some of the analyses of LTCM’s downfall invoke formerly taboo concepts like overbetting and the capital growth criterion to address the question of how much risk is “too much.”
Among the small group of Kelly economists and money managers, the rhetoric is stronger yet. In several articles, portfolio manager Jarrod Wilcox offers a sweeping vision in which overbetting is behind many of the world’s financial ills—not only LTCM but Enron, debt-financed telecommunications industry overexpansion, and the 1987 failure of portfolio insurance on Black Monday. In a 2003 issue of
Wilmott
magazine, Thorp linked the LTCM collapse to Merton and Scholes’s intellectual critique of the Kelly system: “I could see that they didn’t understand how it controlled the danger of extreme risk and the danger of fat-tail distributions,” Thorp said. “It came back to haunt them in a grand way.”
Could Kelly money management have prevented the LTCM disaster? It is easy to see the appeal of the Kelly philosophy. In a world where return is so highly valued, people will always be tempted to venture out onto the precipice. The Kelly criterion tells
exactly
how far a trader can go before tumbling into the abyss. Mean-variance analysis and VaR do not.
In the most direct human terms, LTCM’s problem was group-think. Under John Meriwether, there was an organizational culture in which questions of risk were pressed only so far. This appears to have led to systematically rosy projections. Too little of the fund’s brainpower went to skeptical probing of what could have gone wrong.
LTCM goofed by greatly underestimating the chance of a panic in which its trades would become highly correlated. The fund was making hundreds of simultaneous bets. It operated on the assumption that these bets had low correlation. The chance of all the bets going bad at once was estimated to be fantastically small. Then Russia defaulted, and suddenly a lot was riding on the same losing hand. LTCM had “a whole lot of bets on Southeast Asian debt, a whole lot of bets on the spread between government and junk,” Thorp said. “So it’s not really millions of small bets. It’s a few big bets.”
You might then ask how LTCM would have been any better off with the Kelly system. The answer is that the Kelly criterion can be more forgiving of human error than many other systems—including highly leveraged approaches such as LTCM’s. Recall the example of simultaneous bets on a large number of coins, each with a 55 percent chance of coming up heads. The Kelly bettor stakes
almost
his whole bankroll, splitting the wagered money equally among all the coins. He refuses to bet the entire bankroll because of the remote chance that every single coin will come up tails.
This illustrates the “paranoid” conservatism of Kelly betting. The chance of hundreds of coins simultaneously coming up tails is of course astronomically small. No matter—the ideal Kelly gambler’s “survival motive” precludes taking any chance of ruin whatsoever. By not betting the entire bankroll, the Kelly bettor is taking out an “insurance policy” guaranteeing that he will be able to recover after any possible run of bad luck.
It is easy to do better than the Kelly gambler in the short run. Someone who skips the “insurance” and bets 100 percent of her bankroll, spreading it among the hundreds of simultaneous favorable bets, is not likely to have cause to regret it anytime soon. And why stop there? You can be more aggressive by using leverage. Borrow twenty-nine times your bankroll, add it to your own money, and apportion it among all the coins. You will make thirty times the profit, on average.
The downside is that there is a chance of losing everything, and a further chance of ending up in debt to your lenders. These chances are not quite so remote. When you use leverage, you have to get a certain number of winning tosses just to pay back your lenders. If you don’t get them, you’re broke or in debt.
Are these chances acceptable? You can do a VaR calculation to help decide. Pick a leverage and a risk level that feels right, and go for it.
This is roughly what LTCM did. It is not necessarily crazy. We all take risks that are inconsistent with living forever. But this approach leaves little margin for error.
Estimates about the market’s probabilities are always going to be just that: estimates. It is good practice to have a sense of how far off these estimates may be, and how much likely errors would affect the results. “Margins of error” are themselves estimates. Human nature often skews these estimates optimistically.
A decade rarely passes without a market event that some respected economist claims, with a straight face, to be a perfect storm, a ten-sigma event, or a catastrophe so fantastically improbable that it should not have been expected to occur in the entire history of the universe from the big bang onward. In a world where financial models can be so incredibly wrong, the extreme downside caution of Kelly betting is hardly out of place. For reasons mathematical, psychological, and sociological, it is a good idea to use a money management system that is relatively forgiving of estimation errors.
Fat Tails and Leverage

Suppose you’re betting on a simultaneous toss of coins believed to have a 55 percent chance of coming up heads, as depicted on the previous page. But on this toss, only 45 percent of the coins are heads. Call it a “fat tail” event, or a failure of correlation coefficients, or a big dumb mistake in somebody’s computer model. What then?
The Kelly bettor cannot be ruined in a single toss. (He is prepared to survive the worst-case scenario, of
zero
heads.) In this situation, with many coins, the Kelly bettor will stake just short of his full bankroll. He wins only 45 percent of the wagers, doubling the amount bet on each coin that comes up heads. The Kelly bettor therefore preserves at least 90 percent of his bankroll.
If the preponderance of tails on this toss is just bad luck, the Kelly bettor can expect to recover lost ground on succeeding tosses. If instead the “real” chances are less favorable than the estimated 55 percent, the would-be Kelly bettor will actually be overbetting. This will cut into compound return and increase volatility. At any rate, the Kelly bettor will have time to live and learn, revising probability estimates along the way.
Compare this to someone who uses thirty-times leverage. Instead of losing just 10 percent of the bankroll, the leveraged bettor loses 300 percent. That means he loses everything and still owes twice the amount of the previous bankroll to lenders. He probably can’t learn from this mistake, either. Who’s going to give him another chance?
The core of John Kelly’s philosophy of risk can be stated without math. It is that even unlikely events must come to pass eventually. Therefore, anyone who accepts small risks of losing everything
will
lose everything, sooner or later. The ultimate compound return rate is acutely sensitive to fat tails.
The University of British Columbia’s William Ziemba has estimated that LTCM’s leverage was somewhere around twice the Kelly level. If correct, that would imply that the fund’s true compound growth rate was hovering near
zero
.
The familiar mean-variance mapping is not a good way of visualizing this type of problem, noted the University of North Carolina’s Richard McEnally. In the mean-variance mapping (left), return rises as a straight line as leverage increases. Risk rises, too, but this diagram shows no reason why a very aggressive and risk-tolerant trader should
not
increase leverage to any degree obtainable. In the Kelly mapping (right), the line of return is a curve that boomerangs back to zero and negative returns.
Two Views of Risk and Return
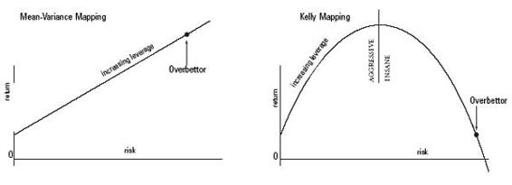
It is not a question of which mapping is “right.” Both mappings are right for different contexts. The highly leveraged overbettor is likely to do well on many bets that are not parlayed. It is when bets compound over time that the Kelly mapping becomes all-important. A strategy like LTCM’s fails—and here the fund’s name is grimly ironic—in the long term.
For true long-term investors, the Kelly criterion is the boundary between aggressive and insane risk-taking. Like most boundaries, it is an invisible line. You can be standing right on it, and you won’t see a neat dotted line painted on the ground. Nothing dramatic happens when you cross the line. Yet the situation on the ground is treacherous because the risk-taker, though heading for doom, is liable to find things getting better before they get worse.
“Convergence trades are a real snake pit,” said Thorp, “unless they have a timetable driving them, such as an expiration date in the case of warrants, options and convertible bonds.” LTCM was trading thirty-year bonds. It was in no position to wait thirty years for “sure” profits. Nor could it have reduced leverage on these trades, with their tiny profit margins, and remained attractive to investors. “If they had not overbet,” noted Thorp, “it seems likely that, with a 0.67 percent expected gain (annualized) on a typical trade, leverage of, say, 5 or 10 would only produce gains of 3.3 to 6.7 percent—hardly interesting to the general partners or investors.” By comparison, had LTCM skipped the fancy arbitrage and simply bought thirty-year Treasury bonds at August 1998 rates, it would have earned a rock-solid 5.54 percent.