The Return of Vaman - A Scientific Novel (10 page)

Arul was deeply engrossed in what he was reading. John von Neumann’s thesis in ‘Theory of self-reproducing automata’ had taken hold of him. Extensive commentaries by experts accompanied the original work. He had first heard of this famous mathematician at school. His maths teacher had set the class a tricky problem: Two railway stations are 120 miles apart. Two trains start at the same time from the two stations and move towards each other along the same tracks. One has the speed of 20 miles per hour while the other moves at 40 m.p.h. A fly that was sitting on the engine of the first train travels at the speed of 50 m.p.h. until it lands on the engine of the second train, whence it rebounds and flies back towards the first train. It keeps shuttling between the trains at the same speed until the engines collide and the fly is crushed to death. How many miles did the fly traverse during its entire journey?

Arul had solved that problem with relative ease. He figured out that the distance between the two trains was steadily diminishing at the rate of 20 + 40 = 60 m.p.h. For them to collide, this distance (which was originally 120 miles) must reduce to zero. This would take two hours to happen. In those two hours the fly would have travelled altogether 2 × 50 = 100 miles.

‘Well done!’ the teacher had said. ‘Do you know how von Neumann, the famous mathematician solved this problem?’ The teacher related the tale of how a friend posed the problem to von Neumann, who had almost instantly given the right answer. ‘How did you solve it?’ the friend asked. ‘Why, there is only one method that I know of—the direct one’, von Neumann elaborated. ‘You calculate all the distances the fly covered from engine to engine. She made infinitely many trips to and fro, but the distance in each trip is reduced in a geometric progression. I summed the series and gave you the answer.’

This direct method requires considerably more calculation, and a reasonably bright mathematician can do the sum in about ten to fifteen minutes. That von Neumann got the answer so fast was typical of how rapidly his brain functioned. To Arul it was more revealing in the sense that his method was the one a computer would use to solve the problem. The direct method—it may involve more calculations—but time was no problem to a fast computer. So von Neumann’s brain was ideally suited to think about how a computer would work. No wonder he had proposed the notion of this fantastic machine.

As von Neumann found, there is a basic difficulty in fabricating an intricate machine. For it demands a complicated system of logic which needs a highly elaborate set of cross connections for transmitting information. And the more complicated the logic, the easier it is to make mistakes. From hand-operated machines to automatic ones, the complexity and the likelihood of breakdown both increase. A self-reproducing automaton is of necessity far more complex than an ordinary one.

Realizing this difficulty and the near impossibility of achieving foolproof logic, von Neumann introduced what he called ‘probabilistic logic’. It was a system of logic applied to an intricate device, not all the components of which were expected to function correctly at all times. The probability of breakdown of some logical step was thus allowed for.

Based on such a system of logic, von Neumann had proved many mathematical theorems. Some of his conclusions went beyond abstract notions, for he offered explicit constructions for realizing them in practice. A machine with considerable complexity of automation may be able to make another with lesser complexity. But can it make a copy of itself? ‘Yes’, said von Neumann, and proved it mathematically. His construction of such an automaton was, however, too complicated to be within the scope of current technology. At least so the experts thought, and Arul agreed with them.

But what about future, more advanced technologies? If they could make a self-reproducing automaton, what problems would they pose? Von Neumann’s work raised such questions. Would these machines follow the evolutionary doctrine applicable to living systems, wherein species improve through natural selection and interaction with the environment? Can one generation of automaton ‘learn’ from the mistakes of the previous generation?

To Arul one question kept coming back again and again. If the automata strove to improve themselves at all costs, how would they deal with anything that interfered with that goal—even assuming that the interfering agency was human?

‘Laxman, at your suggestion, I studied von Neumann’s thesis. I now appreciate the enormity of the problem you are trying to solve. Without the blessings of the Guru, I would say that your task is an impossible one’, Arul said.

‘For me, the main work has already been done by those who left the container behind. I am merely trying to understand their prescription. Even that is proving hard enough’, Laxman replied.

‘Ha! Ha! It’s very amusing to see you being reduced to a computer that follows programmed instructions.’

‘Not even that! The computer at least understands the instructions. I have yet to reach that stage. Which is where Guru helps me.’ And Laxman pointed to a locker.

The sanctum sanctorum of the computer room was accessible only to Arul and Laxman. Strict security, manual and automatic, ensured that nobody else could enter. Even in such a guarded place Laxman had a specially made locker.

‘Over there, further instructions are to be fed to the robot I am making. According to Guru, the information has to be fed in a certain order to be strictly adhered to.’

‘That is fine so far as it goes! But Laxman, I have a word of caution. You made Guru as per the instructions. So far so good. We know what computers are. This one is highly exceptional, but still not totally unfamiliar to us. But what you are making now is new … alien.’

‘Bah! You are probably reading too much sci-fi, Arul. Alien, indeed! This robot is after all a robot, an automaton. One that can be controlled from without—not Frankenstein’s monster.’ Laxman laughed.

‘Nevertheless, I must advise caution. Don’t get carried away.’ Arul’s face expressed genuine worry.

‘OK! Let me promise to consult you at each important step in the project. How is that?’ Laxman asked, still in a light vein.

‘Good, but not good enough. We need an experienced mind. I would greatly value views from Professor Kirtikar. We consulted him in the beginning, remember?’

‘Done, Arul, done. To hear you speak, I am beginning to feel as though I am making a nuclear bomb instead of Vaman.’

‘Vaman?’ Arul was hearing the name for the first time.

‘My projected robot. I have already named him Vaman—for the instructions as interpreted by Guru make him only about a metre tall. What do you think of the name?’

Vaman! One of the ten incarnations of Vishnu when he appeared as the Dwarf God.

‘Appropriate … very appropriate’, said Arul thoughtfully. The name had other connotations that worried him momentarily. According to the Hindu legend, King Bali offered Vaman as much space as he could cover in three steps. Thereupon Vaman grew, and grew, so that his three steps covered the heavens and all of Earth, with the result that Bali was deprived of his entire kingdom and had to retreat to the underworld. Would Laxman’s Vaman develop sinister intentions? Arul had vague misgivings, but he dismissed them as he nodded heartily and said:

‘Three cheers for Vaman!’

© Springer International Publishing Switzerland 2015

 

Laxman thought that even Guru would not be able to cope with the complexity of instructions needed for the making of Vaman as, once again, he encountered the verdict ‘Programme Error’ on his terminal. But from past experience he had learnt to respect Guru. Previous programme errors had turned out to be traceable to his own mistaken execution of Guru’s instructions. On each occasion Guru had correctly interpreted the Vaman code, as Laxman called it.

The complexity had increased as he progressed. There were occasions when he felt like giving up, but the tantalizing goal egged him on. That he was making a robot was now known all over the Science Centre. Like Guru, the robot was expected to be exceptional. But nobody knew in what way—certainly no one even imagined that a thinking, self-replicating robot was in the making.

‘How long before we may expect the incarnation of Vaman?’ asked Navin on one of those frustrating days.

‘A couple of weeks, at least. The hardware problems of his brain still need to be sorted out’, Laxman answered.

‘But how about your own work, Navin?’ Arul asked, knowing full well that this was the only way of diverting Navin from his questions.

He was right. Navin was at heart a research worker. He relished narrating how he had deciphered archaeological clues to reconstruct an era that was long past. As Navin embarked on his elaborations, Laxman broke in: ‘Navin! You have so much to say. Why limit it to canteen table talk? It is about time you addressed the entire staff of the Science Centre on all your finds.’

‘Absolutely!’ said Navin. ‘I am very keen to give a detailed talk so that I can share my excitement with others. I will do so once all my slides are made.’ And he rushed off on his errand.

‘Navin has changed’, Arul commented as his figure disappeared through the door.

‘Yes. The Major’s medicine has worked. He has decided to play ball. He has been dutifully conveying my false data on Guru’s CPU to Pyarelal. The Major threatened him with god knows what, but he has turned the corner’, Laxman replied.

‘I wonder what our friend Yamamoto will say once he realizes that he has been duped’, Arul mused.

‘It is not as simple as that, Arul’, Laxman smiled, for he was about to reveal his secret. ‘The information is so complex that it will take Yamamoto and his bunch of experts a considerable period to realize that it is basically defective. They will continue thinking that their implementation is at fault. And meanwhile, they have no option but to rely on what we supply them with. Indeed, Samant is hopeful that once Vaman’s reputation gets about, Shulz will pay us a visit … and walk right into a well-laid trap.’

‘But what is to prevent Shulz from operating from afar—he need not enter India. He has that agent of his, Pyarelal’, Arul retorted.

‘Samant does not think so, and I agree with him’, Laxman said. ‘Vaman will be a prize that Shulz cannot entrust to others—it is too important to him for that. It will be the supreme achievement of his nefarious career. Vaman will be the bait for Shulz.’

‘I hope you’re right, Laxman. I hope, too, that Navin has really turned over a new leaf. It is a dangerous game that Samant is playing’, Arul said.

‘May be you are right. In any case it is necessary for both of us to be very cautious. But as far as strategy is concerned, I leave it to the experts. Samant evidently knows what he is up to. Well, I must be on my way back to the drawing board, Arul.’

Laxman got up, but Arul remained seated, a thoughtful expression on his face.

The auditorium of the Science Center was packed to capacity. This was the occasion when the staff was to be briefed about the contents of the box which had started all the activity. Exactly at 5 p.m., Navin rose to give his presentation. He had come armed with slides and viewgraphs.

‘Friends,’ he began in a voice scarcely concealing his excitement, ‘this container at Gauribidnur is without doubt the greatest archaeological find ever. Of course, you are dazzled by the supercomputer Guru and looking forward to the arrival of Vaman. The technological fallout of the container is indeed fantastic, but to a person like me the past holds all the allure. So let me take you back to the ancient times when the people who left the container behind lived and flourished …

‘I was intrigued by them right from the day I set my eyes on the container. I will not bore you with technicalities, about the methods commonly used by archaeologists to interpret the relics they find. Instead I will give you the final outcome of my investigations in ready-made form. Those who want to know the why and wherefore of it may reserve their questions to the end.

‘I estimate that this civilization is about twenty thousand years old. All our so-called ancient civilizations—of Egypt, Babylon, China, or Harappa-Mohenjodaro—are modern compared to this one. People argue about the exact time of the Vedas. But even with the oldest quoted estimate, the Vedic era occurred much later than this civilization.

‘These people called themselves Monads, after Mona, the Earth in their language. It was a worldwide culture, transcending regional differences of geography. There were no nation-states, nor were there any tensions or quarrels between different regions. It was truly one family of people inhabiting our planet.

‘There are two ways of estimating how advanced a civilization is. One method uses the measure of energy consumed by a civilization, not only to maintain its standard of living but also in the exchange and broadcast of information. On this scale, the Monads were a thousand times more advanced than we are now. For comparison, the gap between the Greek civilization of two millennia ago and us is about the same.

‘The second method estimates the total information content of the civilization in question. Knowledge
per se
can of course defy objective evaluation, but the language in which it is expressed lends itself to quantification. For those unfamiliar with computers and binary arithmetic, I will digress a little to explain this point.’

Navin paused to sip some water and then projected his first transparency on the screen. It showed positive (+) and negative (–) signs distributed in thirty-two rows, with five signs in each row:

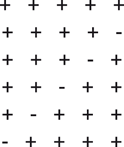
He then continued.

‘On the screen you see thirty-two different alternatives for distributing pluses and minuses in five places. Why thirty-two? The answer is simple. Each of the five places has two alternatives, either a plus or a minus. Since each alternative for the first place can be combined with each alternative for the second and so on, there are in all 2 × 2 × 2 × 2 × 2 = 32 ways of arranging these signs.

‘What has all this to do with information, you may ask. Well, in a computer’s binary logic, the basic information consists of the alternatives ‘yes’ or ‘no’ to each question. These are my plus and minus signs. Each set of alternatives is called an information bit. What you see on the screen are the thirty-two different sets of alternatives of information available through five bits.

‘Now we can identify each of the quintets with a letter in the English alphabet together with six signs like the full stop, comma, etc. A four-letter word will thus need twenty information bits, and a ten-word sentence of forty letters, two hundred bits. A book of fifty thousand words will need approximately one million bits.

‘Let us estimate the total number of different books in the English language as around ten million, based on the inventories of the biggest libraries in the world. Their information content is around ten million million bits. Taking into account books in other languages and also the fact that other modes like pictures and music also carry information, the total information content of our civilization is no more than a million billion bits.

‘Again, this is about a thousand times more than what the Greeks had. The Monads, on the other hand, were a thousand times better informed than us!

‘However, their numbers were limited—no more than around a hundred million. Therein lay the cause of their prosperity. They could and did control their population and used the resources of our planet judiciously. Their colonies were typically of twenty to thirty thousand people. Their main energy sources were two: the direct exploitation of solar energy and the fusion reactors that our scientists are striving to construct today.

‘With this introduction I now show you the slides I got specially made based on the information supplied by the container. I will also give some factual information on the transparencies.’

So for the next hour Navin held forth brilliantly. His talk was followed by numerous questions, the last of which came from Arul: ‘Why did they bury this time capsule in Gauribidnur, of all places?’

‘As far as I can make out, this place was an important administrative centre for the Monads, one of the ten dotted all over the globe. They wanted to choose a site near an administrative centre and fulfilling certain conditions. The place had to be free from earthquakes, as this one is. The rock strata here are remarkably stable. So anything buried here would remain undisturbed. The Japanese location, for example, did not meet this criterion. Next, they wanted the underground water table to be very low—which ruled out their European centre near Holland. A third criterion was that the container should be well away from the seashore—satisfied here, but not by their centre in what we today call Florida … Well, the long and the short of it is that, after these and many other considerations, our spot right here turned out to be the best.’

‘Ah, that explains it’, Arul replied after Navin had finished.

‘Explains what?’ Navin asked.

‘Why did we discover the container here? My criteria for housing the gravity experiment were precisely these! On the basis of these I chose Gauribidnur as the best site. So our digging here and finding the container was not as great a coincidence as I had imagined’, Arul said.

After Navin’s talk, Arul and Laxman went over to the latter’s living quarters. Urmila joined them shortly afterwards.

‘And where have you been my little maid?’ Laxman asked.

‘Why, to the lecture, of course. Wasn’t it magnificent? Now I know why Navinji is so much in demand as a speaker. He can feel the pulse of the audience—what a contrast from my dear hubby’, Urmila answered, squeezing Laxman’s hand.

‘Laxman, it looks as if your talents are not appreciated at home’, Arul added jocularly.

‘I gave up lecturing to her long ago. She can’t understand even the simplest things on computers’, Laxman said.

‘Arul, you should ask your friend what he means by the “simplest”. His idea is to quote some obscure algorithm to start with and follow it up with totally incomprehensible questions’, Urmila put her case.

‘Well Madam, here is a simple question which even you can comprehend: when are we to get our coffee?’

‘I am like your computer, sir—I can execute orders only after you have given them. So you will have to wait fifteen minutes.’

As Urmila went into the kitchen, Laxman put on an LP of Beethoven’s fifth symphony. Urmila’s return with the coffee brought the two scientists out of the respective reveries which the profound music had plunged them into.

‘Arul, perhaps you can answer my question in simpler language than my husband can’, Urmila asked, as they sipped the delicious ‘real’ coffee that no ‘instant’ version can ever emulate.

‘Go ahead, I will try’, Arul replied.

‘Navinji did not make one point clear … Why did the Monadic civilization, which was so advanced, become extinct?’

Before Arul could reply Laxman broke in: ‘Umi, your question is basically illogical. Navin’s talk was based on what he found in the container. Evidently, whatever was in the container was left by the Monads when they were alive and flourishing. How can you expect to know from that how they died? It is as ludicrous as expecting a person to write his own obituary.’

‘Arul, here you see an example of Laxman’s obscurantism.’

Arul, however, was thoughtful as he replied slowly. ‘Urmila, Laxman’s technical objection apart, your question is basically a valid one, and worrying at that. In the accounts found in the container we see a picture of a well run society. Evidently the Monads had to face some unexpected natural catastrophe. What could it have been? An ice age? A major earthquake? Or a volcanic eruption? Did a meteorite or a comet hit Earth? It had to be something that could not be overcome by the Monads with all their very advanced technology and meticulous planning.’

Laxman shook his head in disagreement. ‘Talk sense, Arul. Natural disasters can be catastrophic, I admit that. But a really big event like that is bound to leave a mark or two behind. Moreover, twenty thousand years is not really long enough to obliterate those marks. Why don’t we see any relics today?’

Laxman’s point was well taken. Arul had no answer. Why were the Monads wiped off the face of the Earth? The container, as Laxman had argued, would not be expected to provide the answer. How and where would they find it?