World's 200 Hardest Brain Teasers (4 page)
Read World's 200 Hardest Brain Teasers Online
Authors: Dr. Gary R. Gruber

100. Refrigerators come in cartons 40 inches deep x 48 inches wide x 60 inches high. They must stand upright when stored. If Jones has a storage room 45 feet across, 60 feet deep, and 8 feet high, what is the greatest number of refrigerators he can store there?
(a) 180
(b) 195
(c) 198
(d) 201
(e) 396
101. Which is greater, Quantity A or Quantity B? Or are they equal?
QUANTITY A: The average rate of a car traveling uphill at a rate of
a
miles an hour and downhill the same distance at a rate of
b
miles an hour.
QUANTITY B: The average of the rates of
a
miles an hour and
b
miles an hour.
102. What can we infer from the following statement?
“Since every child I know likes ice cream, Mike must also like ice cream.”
(a) The speaker doesn’t know many children.
(b) Mike is a child.
(c) Mike likes anything sweet.
(d) The speaker is a good friend of Mike’s.
(e) The speaker saw Mike eat ice cream.
103. What word would become smaller when you add additional letters?
104. Do they have a fourth of July in England?
105. How many birthdays does the average man have?
106. How many outs are there in an inning?
107. A clerk in the butcher shop is 5'10” tall. What does he weigh?
108. The three symbols in sets I and II have something in common. What must the “?” be so that an analogy exists between the first and second set?
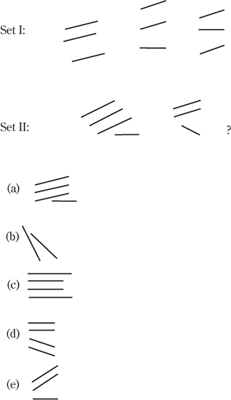
109. How many two-cent stamps are there in a dozen?
110. If a doctor gives you three pills, telling you to take one every half hour, how many minutes will pass from taking the first pill to the last pill?
111. One of the numbers between 3,000 and 4,000 with digits increasing from left to right is 3,457. How many different numbers are there with digits that increase from left to right between 4,000 and 5,000?
112. What is the next number in the following sequence: 125, 64, 27, 8 ?
113. John is 10 years old, and his mother is four times as old as he is. When John is 15 years old, how old will his mother be? (Assuming she is not dead.)
(a) 65
(b) 60
(c) 55
(d) 50
(e) 45
114. Suppose a car goes uphill a distance of 1 mile, then immediately turns around and goes downhill the same distance, and suppose the average rate of the car for the whole trip is 20 miles per hour. What is the total time spent going uphill and downhill in minutes?
(a) 6
(b) 8
(c) 10
(d) cannot be determined unless the time going downhill is given
(e) cannot be determined unless the speed going uphill is given
115. If you are in an elevator stopped on the thirty-ninth floor of a hotel and the cable breaks and no help is available, what do you do to save yourself?
(a) Just before the elevator hits the ground, you jump up.
(b) Hold on to anything and stay still.
(c) Lie down flat in the elevator.
(d) You can’t do anything.
116. Is 46 x 767 x 72 greater, less than, or equal to 767 x 46 x 74? You have three seconds to answer.
117. Create a sentence such that when “which” is replaced by “that” and the commas are inserted in the appropriate places, the sentence has a different meaning.
118. Nine people are on a straight line inside a circle. What is the least number of people that must move from the line in order for all nine people to be on the circumference of the circle?
119. In five seconds or less, which is greater: 1 or 77/99 divided by 99/77?
120. Fill in the blanks to complete the sentence: While a television course is not able to________a live course, it is still able to teach the________aspects of the subject.
(a) develop…necessary
(b) replace…important
(c) manage…relevant
(d) create…negative
(e) anticipate…inconsequential
121. What is the main problem with the following argument? “Women are better than men in tennis. It is true that Bobby (Robert) Riggs beat Margaret Court, but he played like a woman and she played like a man.”
122. VACCINATION : DISEASE ::
(a) aspirin : headache
(b) studying : learning
(c) physician : patient
(d) trial : judgment
(e) freezing : spoilage
123. Mary must get up at 7 AM to get to work on time. Her clock gains 9 minutes every 3 days. If she sets it correctly at 11 PM on Sunday night, at what time should she get up, according to her clock, on Tuesday morning?
124. Phil is taking a 100-mile trip. If he averages 25 miles per hour during the first 50 miles, what must he average during the second 50 miles to make his average speed for the whole trip 50 miles per hour?
125. There are nine coins that are identical in appearance. One weighs more than the others, which have equal weight. With a balance scale to determine the coin that is heavier in only two weighings, how many coins on each side of the balance scale would you weigh first?
(a) 1 vs. 1
(b) 2 vs. 2
(c) 3 vs. 3
(d) 4 vs. 4
(e) none of these
126. One segment of the game show
Let’s Make a Deal
had three doors—behind one door was a new car and behind each of the other two was a goat. The contestant would win whatever was behind the door he or she chose. The contestant chose one of the three doors, but before it was opened, the host opened up a different door that had a goat behind it. In order to have the greatest chance of winning the car, should the contestant open the door that was his or her original choice or open the remaining door?
127. The following words were in a sentence in the following sequence: XYere ZYere Xere. What letters do X, Y, and Z represent?
128. Suppose you can purchase donuts in boxes of 6, 9, and 20. What is the greatest number of donuts you cannot purchase?
129. In 10 seconds, with or without a calculator, what is the value of 2/3 x 3/4 x 4/5 x 5/6 x 6/7?
130. LIAR : MENDACIOUS ::
(a) disease : toxic
(b) artist : creative
(c) conductor : symphonic
(d) pygmy : undersized
(e) fib : concerned
131. What is the next letter in the following series: a l b e m f i n j ?
(a) l
(b) m
(c) o
(d) p
(e) q
132. Suppose you have three Scrabble tiles: N, T, and O. What is the probability that when you randomly place the three tiles upright in a row, they spell an English word?
133. What is the value of the sum of the first 99 consecutive integers? (In other words, what is 1 + 2 + 3 + 4 +…+ 99?)
134. Suppose we have the sequence (1), (2,3), (4,5,6), (7,8,9,10), (11,12,13,14,15)…where (1) is the first part of the sequence (2,3) the second part, etc. What is the first number of the 100th part of the sequence?
135. In an isosceles triangle, ABC, AB = AC, and angle A = 20°. Point P is on side AC such that AP = BC. Find angle PBC.

136. P is a point inside a square ABCD such that PA = 1, PB = 2, and PC = 3. What is the measure of angle APB?
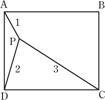
137. Which two words do not belong with the others?
(a) fallible
(b) congruous
(c) flammable
(d) famous
(e) exact
138.

Given: BAC = 20°
BAC = 20° ABC =
ABC = ACB
ACB ABE = 20°
ABE = 20° DCA = 30°
DCA = 30° BED = ?
BED = ?