Against the Gods: The Remarkable Story of Risk (33 page)
Read Against the Gods: The Remarkable Story of Risk Online
Authors: Peter L. Bernstein

But beyond all that, Galton wanted to prove that heredity alone was the source of special talents, not "the nursery, the school, the university, [or] professional careers."30 And heredity did seem to matter, at least within the parameters that Galton laid out. He found, for example, that a ratio of one out of nine close relatives of 286 judges were father, son, or brother to another judge, a ratio far greater than in the general population. Even better, he found that many relatives of judges were also admirals, generals, novelists, poets, and physicians.*
(Galton explicitly excluded clergymen from among the eminent). He was disappointed to note that his "finger marks" failed to distinguish between eminent men and "congenital idiots."31
Yet Galton discovered that eminence does not last long; as physicists would put it, eminence has a short half life. He found that only 36% of the sons of eminent men were themselves eminent; even worse, only 9% of their grandsons made the grade. He attempted to explain why eminent families tend to die out by citing their apparent habit of marrying heiresses. Why blame them? Because heiresses must come from infertile families, he argued; if they had had a large number of siblings with whom to share the family wealth, they would not have inherited enough to be classified as heiresses. This was a surprising suggestion, in view of the comfort in which Galton lived after sharing his father's estate with six other siblings.

After reading Hereditary Genius, Charles Darwin told Galton, "I do not think I ever in my life read anything more interesting and original ... a memorable work."32 Darwin suggested that he go on with his analysis of the statistics of heredity, but Galton needed little encouragement. He was now well on his way to developing the science of eugenics and was eager to discover and preserve what he considered to be the best of humanity. He wanted the best people to have more offspring
and the lowly to exercise restraint.
But the law of the deviation from the mean stood stubbornly in his
way. Somehow he had to explain differences within the normal distribution. He realized that the only way he could do so was to figure out
why the data arranged themselves into a bell curve in the first place.
That search led him to an extraordinary discovery that influences most
of the decisions we make today, both small and large.
Galton reported the first step in an article published in 1875, in
which he suggested that the omnipresent symmetrical distribution around
the mean might be the result of influences that are themselves arrayed
according to a normal distribution, ranging from conditions that are most
infrequent to conditions that are most frequent and then down to a set of
opposite kinds of influences that again are less frequent. Even within
each kind of influence, Galton hypothesized, there would be a similar
range from least powerful to most powerful and then down again to least
powerful. The core of his argument was that "moderate" influences
occur much more often than extreme influences, both good and bad.
Galton demonstrated this idea with a gadget he called the Quincunx
to the Royal Society around 1874.33 The Quincunx looked a lot like an
up-ended pinball machine. It had a narrow neck like an hour-glass, with
about twenty pins stuck into the neck area. At the bottom, where the
Quincunx was at its widest, was a row of little compartments. When
shot were dropped through the neck, they hit the pins at random and
tended to distribute themselves among the compartments in classic
Gaussian fashion-most of them piled up in the middle, with smaller
numbers on either side, and so on in diminishing numbers.
In 1877, in conjunction with his reading of a major paper titled
"Typical Laws of Heredity," Galton introduced a new model of the
Quincunx. (We do not know whether he actually built one). This
model contained a set of compartments part way down, into which the
shot fell and arrayed themselves as they had in the bottom compartments in the first model. When any one of these midway compartments
was opened, the shot that had landed in it fell into the bottom compartments where they arrayed themselves-you guessed it-in the usual
normal distribution.
The discovery was momentous. Every group, no matter how small
and no matter how distinct from some other group, tends to array itself in accordance with the normal distribution, with most of the observations landing in the center, or, to use the more familiar expression, on
the average. When all the groups are merged into one, as Quincunx I
demonstrated, the shot also array themselves into a normal distribution.
The grand normal, therefore, is an average of the averages of the small
subgroups.
Quincunx II provided a mechanical version of an idea that Galton
had discovered in the course of an experiment proposed by Darwin in
1875. That experiment did not involve dice, stars, or even human
beings. It was sweet peas-or peas in the pod. Sweet peas are hardy and
prolific, with little tendency to cross-fertilize. The peas in each pod are
essentially uniform in size. After weighing and measuring thousands of
sweet peas, Galton sent ten specimens of each of seven different weights
to nine friends, including Darwin, throughout the British Isles, with
instructions to plant them under carefully specified conditions.
After analyzing the results, Galton reported that the offspring of the
seven different groups had arrayed themselves, by weight, precisely as
the Quincunx would have predicted. The offspring of each individual
set of specimens were normally distributed, and the offspring of each of
the seven major groups were normally distributed as well. This powerful result, he claimed, was not the consequence of "petty influences in
various combinations" (Galton's italics). Rather, "[T]he processes of
heredity ... [were] not petty influences, but very important ones."34
Since few individuals within a group of humans are eminent, few of
their offspring will be eminent; and since most people are average, their
offspring will be average. Mediocrity always outnumbers talent. The
sequence of small-large-small distributions among the sweet peasaccording to the normal distribution-confirmed for Galton the dominance of parentage in determining the character of offspring.
The experiment revealed something else, as the accompanying
table of diameters of the parent peas and their offspring shows.
Note that the spread of diameters among the parents was wider
than the dispersion among the offspring. The average diameter of the
parents was 0.18 inches within a range of 0.15 to 0.21 inches, or 0.03
on either side of the mean. The average diameter of the offspring was
0.163 inches within a range of 0.154 to 0.173 inches, or only about
0.01 inches on either side of the mean. The offspring had an overall
distribution that was tighter than the distribution of the parents.
This experiment led Galton to propound a general principle that
has come to be known as regression, or reversion, to the mean: "Reversion," he wrote, "is the tendency of the ideal mean filial type to
depart from the parental type, reverting to what may be roughly and
perhaps fairly described as the average ancestral type."36 If this narrowing process were not at work-if large peas produced ever-larger offspring and if small peas produced ever-smaller offspring-the world
would consist of nothing but midgets and giants. Nature would become
freakier and freakier with every generation, going completely haywire
or running out to extremes we cannot even conceive of.
Galton summarized the results in one of his most eloquent and dramatic paragraphs:
The child inherits partly from his parents, partly from his ancestry.
... [T]he further his genealogy goes back, the more numerous and
varied will his ancestry become, until they cease to differ from any
equally numerous sample taken at haphazard from the race at large.
... This law tells heavily against the full hereditary transmission of
any gift.... The law is even-handed; it levies the same successiontax on the transmission of badness as well as of goodness. If it discourages the extravagant expectations of gifted parents that their
children will inherit all their powers, it no less discountenances
extravagant fears that they will inherit all their weaknesses and diseases.37
This was bad news for Galton, no matter how elegantly he articulated it, but it spurred him on in his efforts to promote eugenics. The
obvious solution was to maximize the influence of "the average ancestral type" by restricting the production of offspring at the low end of the
scale, thereby reducing the left-hand portion of the normal distribution.
Galton found further confirmation of regression to the mean in an
experiment that he reported in 1885, on the occasion of his election to the presidency of the British Association for the Advancement of
Science. For this experiment, he had gathered an enormous amount of
data on humans, data that he had received in response to a public appeal
backed by an offer of cash. He ended up with observations for 928
adult children born of 205 pairs of parents.
Galton's focus in this case was on height, or, in the language of his
times, stature. His goal was similar to that in the sweet-pea experiment,
which was to see how a particular attribute was passed along by heredity from parents to children. In order to analyze the observations, he
had to adjust for the difference in height between men and women; he
multiplied the female's height in each case by 1.08, summed the heights
of the two parents, and divided by two. He referred to the resulting
entities as "mid-parents." He also had to make sure that there was no
systematic tendency for tall men to marry tall women and for short men
to marry short women; his calculations were "close enough" for him to
assume that there were no such tendencies.38
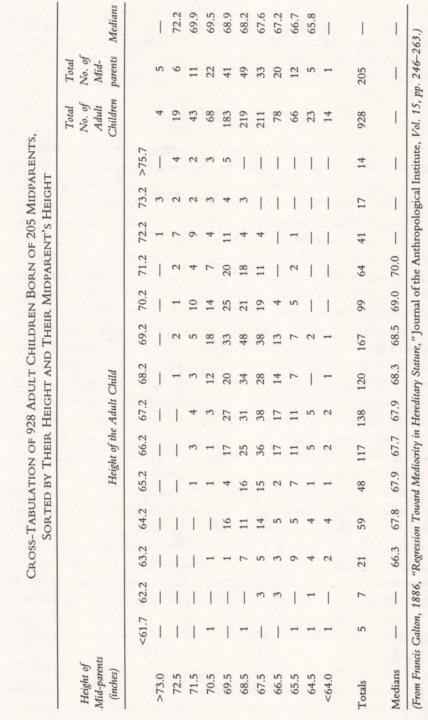
The results were stunning, as the accompanying table reveals. The
diagonal structure of the numbers from lower left to upper right tells us
at once that taller parents had taller children and vice versa-heredity
matters. The clusters of larger numbers toward the center reveal that
each height group among the children was normally distributed and
that each set of children from each parental height group also was normally distributed. Finally, compare the furthest right-hand column to
the furthest left-hand column. ("Median" means that half the group
were taller and half were shorter than the number shown.) The midparents with heights of 68.5 inches and up all had children whose
median heights were below the height of the mid-parents; the mid-parents who were shorter than 68.5 inches all had children who tended to
be taller than they were. Just like the sweet peas.
The consistency of normal distributions and the appearance of regression to the mean enabled Galton to calculate the mathematics of the
process, such as the rate at which the tallest parents tend to produce
children that are tall relative to their peers but shorter relative to their
parents. When a professional mathematician confirmed his results,
Galton wrote, "I never felt such a glow of loyalty and respect towards
the sovereignty and magnificent sway of mathematical analysis."39
Galton's line of analysis led ultimately to the concept of correlation,
which is a measurement of how closely any two series vary relative to one another, whether it be size of parent and child, rainfall and crops,
inflation and interest rates, or the stock prices of General Motors and
Biogen.