The Clockwork Universe (12 page)

Chapter Twenty
The Parade of the Horribles

When Galileo and Newton looked at nature, they saw simplicity. That was, they declared, God's telltale signature. When their biologist colleagues looked at nature, they saw endless variety. That was, they announced, God's telltale signature.

Each side happily cited one example after another. The physicists pointed out that as the planets circle the sun, for instance, they all travel in the same direction and in the same plane. The biologists presented their own eloquent case, notably in a large and acclaimed book titled
The Wisdom of God Manifested in the Works of Creation.
The “vast Multitude of different Sorts of Creatures” testified to God's merits, the naturalist John Ray argued, just as it would show more skill in a manufacturer if he could fashion not simply one product but “Clocks and Watches, and Pumps, and Mills and [Grenades] and Rockets.”

Strikingly, no one saw any contradiction in the views of the two camps. In part this reflected a division of labor. The physicists focused on the elegance of God's aesthetics, the biologists on the range of His inventiveness. Both sides were bound by the shared conviction, deeper than any possible division, that God had designed every feature of the universe. For the physicists, that view led directly to the idea that God was a mathematician, and progress. For biologists, it led down a blind alley and made the discovery of evolution impossible.

Two centuries passed between Newton's theory of gravity and Darwin's theory of evolution. How could that be? Newton's work bristled with mathematics and focused on remote, unfamiliar objects like planets and comets. Darwin's theory of evolution dealt in ordinary words with ordinary things like pigeons and barnacles. “How extremely stupid not to have thought of that!” Thomas Huxley famously grumbled after first reading Darwin's
Origin of Species.
No one ever scolded himself for not beating Newton to the
Principia.

The “easier” theory proved harder to find because it required abandoning the idea of God the designer. Newton and his contemporaries never for a moment considered rejecting the notion of design. The premise at the heart of evolution is that living creatures have inborn, random differences; some of those random variations happen to provide an advantage in the struggle for life, and nature favors those variations. That focus on randomness was unthinkable in the seventeenth century. Even Voltaire, the greatest skeptic of his day, took for granted that where there was a design, there was a designer. No thinker of that age, no matter how brilliant, could imagine an alternative. “It is natural to admit the existence of a God as soon as one opens one's eyes,” Voltaire wrote. “It is by virtue of an admirable art that all the planets dance round the sun. Animals, vegetables, minerals—everything is ordered with proportion, number, movement. Nobody can doubt that a painted landscape or drawn animals are works of skilled artists. Could copies possibly spring from an intelligence and the originals not?”

Newton, blinded by his faith in intelligent design, argued in the same vein. In a world where randomness was a possibility, he scoffed, we'd be beset with every variety of jury-rigged, misshapen creature. “Some kinds of beasts might have had but one eye, some more than two.”

The problem was not simply that for Newton and the others “randomness” conveyed all the horror of “anarchy.” Two related beliefs helped rule out any possibility of a seventeenth-century Darwin. The first was the assumption that every feature of the world had been put there for man's benefit. Every plant, every animal, every rock existed to serve us. The world contained wood, the Cambridge philosopher Henry More explained, because otherwise human houses would have been only “a bigger sort of beehives or birds' nests, made of contemptible sticks and straw and dirty mortar.” It contained metal so that men could assault one another with swords and guns, rather than sticks, as they enjoyed the “glory and pomp” of war.

The second assumption that blinded Newton and his contemporaries to evolution was the idea that the universe was almost brand-new. The Bible put creation at a mere six thou
sand years in the past. Even if someone had conceived of an
evolving natural world, that tiny span of time would not have offered enough elbow room. Small changes could only trans
form one-celled creatures into daffodils and dinosaurs if nature had eons to work with. Instead, seventeenth-century scientists took for granted that trees and fish, men and women, dogs and flowers all appeared full-blown, in precisely the form they have today.

Two hundred years later, scientists still clung to the same idea. In the words of Louis Agassiz, Darwin's great Victorian rival, each species was “a thought of God.”

Chapter Twenty-One
“Shuddering Before the Beautiful”

The seventeenth century's faith that “all things are numbers” originated in ancient Greece, like so much else. The Greek belief in mathematics as nature's secret language began with music, which was seen not as a mere diversion but as a subject for the most intense study. Music was the great exception to the general rule that the Greeks preferred to keep mathematics untainted by any connection with the everyday world
.

Pluck a taut string and it sounds a note. Pluck a second string twice as long as the first, Pythagoras found, and the two notes are an octave apart. Strings whose lengths form other simple ratios, like 3 to 2, sound other harmonious intervals.
²⁷
That insight, the physicist Werner Heisenberg would say thousands of years later, was “one of the truly momentous discoveries in the history of mankind.”

Pythagoras believed, too, that certain numbers had mystical properties. The world was composed of four elements because 4 was a special number. Such notions never lost their hold. Almost a thousand years after Pythagoras, St. Augustine explained that God had created the world in six days because 6 is a “perfect” number. (In other words, 6 can be written as the sum of the numbers that divide into it exactly: 6 = 1 + 2 + 3.)
²⁸

The Greeks felt sure that nature shared their fondness for geometry. Aim a beam of light at a mirror, for example, and it bounces off the mirror at the same angle it made on its incoming path. (Every pool player knows that a ball hit off a cushion follows the same rule.)

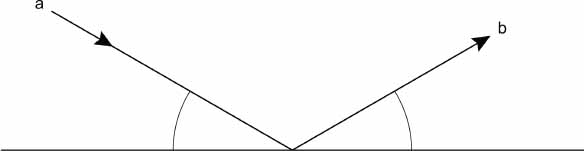
When light bounces off a mirror, the two marked angles are equal.

What looked like a small observation about certain angles turned out to have a big payoff—of the infinitely many paths that the light beam might take on its journey from
a
to a mirror to
b
, the path it actually does take is the shortest one possible. And there's more. Since light travels through the air at a constant speed, the
shortest
of all possible paths is also the
fastest
.

Even if light obeyed a mathematical rule, the rule might have been messy and complicated. But it wasn't. Light operated in the most efficient, least wasteful way possible. This was so even in less straightforward circumstances. Light travels at different speeds in different mediums, for instance, and faster in air than in water. When it passes from one medium to another, it bends.

Look at the drawing below and imagine a lifeguard at
a
rather than a flashlight. If a lifeguard standing on the beach at
a
sees a person drowning at
b
, where should she run into the water? It's tricky, because she's much slower in the water than on land. Should she run straight toward the drowning man? Straight to a point at the water's edge directly in front of the flailing man?

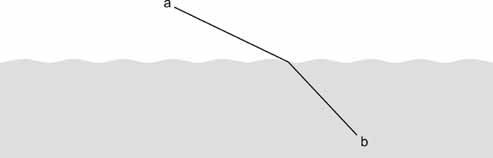
Light bends as it passes from air into water.

Curiously, this riddle isn't in the least tricky for light, which “knows” exactly the quickest path to take. “Light acts like the perfect lifeguard,” physicists say, and over the centuries they've formulated a number of statements about nature's efficiency, not just to do with light but far more generally. The eighteenth-century mathematician who formulated one such principle proclaimed it, in the words of the historian Morris Kline, “the first scientific proof of the existence and wisdom of God.”

Light's remarkable behavior was only one example of the seventeenth century's favorite discovery, that if a mathematical idea
was beautiful it was virtually guaranteed to be useful. Scientists ever since Galileo and Newton have continued to find mysterious mathematical connections in the most unlikely
venues. “You must have felt this, too,” remarked the physicist Werner Heisenberg, in a conversation with Einstein: “the almost frightening simplicity and wholeness of the relationships which nature suddenly spreads out before us and for which none of us was in the least prepared.”

For the mathematically minded, the notion of glimpsing God's plan has always exerted a hypnotic pull. The seduction is twofold. On the one hand, delving into the world's mathematical secrets gives a feeling of having one's hands on nature's beating
heart; on the other, in a world of chaos and disaster, mathematics provides a refuge of eternal, unchallengeable truths and
perfect order.

The intellectual challenge is immense, and the difficulty of the task makes the pursuit even more obsessive. In Vladimir Nabokov's novel
The Defense
, Aleksandr Luzhin is a chess grand master. He speaks of chess in just the way that mathematicians think of their field. While pondering a move and lighting a cigarette, Luzhin accidentally burns his fingers. “The pain immediately passed, but in the fiery gap he had seen something unbearably awesome—the full horror of the abysmal depths of chess. He glanced at the chessboard, and his brain wilted from unprecedented weariness. But the chessmen were pitiless; they held and absorbed him. There was horror in this, but in this also was the sole harmony, for what else exists in the world besides chess?”

Mathematicians and physicists share that passion, and unlike chess players they take for granted that they are grappling with nature's deepest secrets. (The theoretical physicist Subrahmanyan Chandrasekhar, a pioneer in the study of black holes, spoke of “shuddering before the beautiful.”) They sustain themselves through the empty years with the unshakable belief that the answer is out there, waiting to be found. But mathematics is a cruel mistress, indifferent to the suffering of those who would woo her. Only those who themselves have wandered lost, wrote Einstein, know the misery and joy of “the years of searching in the dark for a truth that one feels but cannot express; the intense desire and the alternations of confidence and misgiving, until one breaks through to clarity and understanding.”

The abstract truths that enticed Einstein and his fellow scientists occupy a realm separate from the ordinary world. That gulf between the everyday world and the mathematical one has, many times through the centuries, served as a lure rather than a barrier. When he was a melancholy sixteen-year-old, the modern-day philosopher and mathematician Bertrand Russell recalled many years later, he used to go for solitary walks “to watch the sunset and contemplate suicide. I did not, however, commit suicide, because I wished to know more of mathematics.”

A deep dive into mathematics has special appeal, for it serves at the same time as a way to flee the world and to impose order on it. “Of all escapes from reality,” the mathematician Gian-Carlo Rota observed, “mathematics is the most successful ever. . . . All other escapes—sex, drugs, hobbies, whatever—are ephemeral by comparison.” Mathematicians have withdrawn from the dirty, dangerous world, they believe, and then, by thought alone,
they have added new facts to the world's store of knowledge.
Not just new facts, moreover, but facts that will stand forever,
unchallengeable. “The certainty that [a mathematician's] creations
will endure,” wrote Rota, “renews his confidence as no other
pursuit.” It is heady, seductive business.

Perhaps this accounts for the eagerness of so many seventeenth-century intellectuals to look past the wars and epidemics all around them and instead to focus on the quest for perfect,
abstract order. Johannes Kepler, the great astronomer, barely
escaped the religious battles later dubbed the Thirty Years' War. One close colleague was drawn and quartered and then had his tongue cut out. For a decade his head, impaled on a pike, stood on public display next to the rotting skulls of other “traitors.”

Kepler came from a village in Germany where dozens of women had been burned as witches during his lifetime. His mother was charged with witchcraft and, at age seventy-four, chained and imprisoned while awaiting trial. She had poisoned a neighbor's drink; she had asked a grave digger for her father's skull, to make a drinking goblet; she had bewitched a villager's cattle. Kepler spent six years defending her while finishing work on a book called
The Harmony of the World.
“When the storm rages and the shipwreck of the state threatens,” he wrote, “we can do nothing more worthy than to sink the anchor of our peaceful studies into the ground of eternity.”