The Clockwork Universe (18 page)

Chapter Thirty-One
Two Rocks and a Rope

Tradition has it that Galileo discovered how objects fall by
dropping weights from the top of the Leaning Tower of Pisa. Unlike most legends—Archimedes and his bathtub, Columbus and the flat Earth, George Washington and the cherry tree—
historians believe this one might possibly be true. A tower drop
would have disproved Aristotle's claim that heavy objects fall faster than light ones. But it took the ramp experiments, which Galileo indisputably carried out, to yield the quantitative law about distance and time.

Whether he really climbed a tower or not, Galileo did propose a thought experiment to test Aristotle's claim. Imagine for a moment, said Galileo, that it was true that the heavier the object, the faster its fall. What would happen, he asked, if you tied a small rock and a big rock together, with some slack in the rope that joined them? On the one hand, the tied-together rocks would fall
slower
than the big rock alone, because the small rock would lag behind the big one and bog it down, just as a toddler tied to a sprinter would slow him down. (That was where the slack in the rope came into play.) On the other hand, the tied-together rocks would fall
faster
than the big rock alone, because they constituted a new, heavier “object.”

Which meant, Galileo concluded triumphantly, that Aristotle's assumption led to an absurd conclusion and had to be abandoned. Regardless of what Aristotle had decreed, logic forced us to conclude that all objects fall at the same rate, regardless of their weight. This is a story with a curious twist. Galileo, the great pioneer of experimental science, may never have bothered to perform his most famous experiment. No one is sure. What we know with certainty is that, like the Aristotelians he scorned, Galileo sat in a chair and deduced the workings of the world with no tool but the power of logic.

Since Galileo's day, countless tests have confirmed his Leaning Tower principle (including some at the Leaning Tower itself ). In ordinary circumstances, air resistance complicates the picture—feathers flutter to the ground and arrive long after cannonballs. Not until the invention of the air pump, which came after Galileo's death, could you drop objects in a vacuum. A century after Galileo, the demonstration retained its power to surprise. King George III demanded that his instrument makers arrange a test for him, featuring a feather and a one-guinea coin falling in a vacuum. “In performing the experiment,” one observer wrote, “the young optician provided the feather, the King supplied the guinea and at the conclusion the King complimented the young man on his skill as an experimenter but frugally returned the guinea to his waistcoat pocket.”

Today we've all seen the experiment put to the test, at every Olympic games. When television shows a diver leaping from the ten-meter board, thirty feet above the pool, how does the camera stick with her as she plummets toward the water? Galileo could have solved the riddle—just as a small stone falls at exactly the same rate as a heavy one, a camera falls at exactly the same rate as a diver. The trick is to set up a camera near the diver, at exactly the same height above the water. Attach the camera to a vertical pole and release the camera at the instant the diver starts her fall poolward. Gravity will do the rest.

Galileo exulted in his discovery that “distance is proportional to time squared.” The point was not merely that nature could be described in numbers but that a single, simple law—in operation since the dawn of time but unnoticed until this moment (just as the Pythagorean theorem had been true but unknown before
its
discovery)—applied to the infinite variety of falling objects in the world. A geranium knocked off a windowsill, a painter tumbling off his ladder, a bird shot by a hunter, all fell according to the same mathematical law.

The difference between Galileo's world and Aristotle's leaps out, as we have seen. Galileo had stripped away the details that fascinated Aristotle—the color of the bird's plumage, the motives behind the painter's absentmindedness—and replaced the sensuous, everyday world with an abstract, geometric one in which both a bird and a painter were simply moving dots tracing a trajectory against the sky. Ever since, we have been torn
between celebrating the bounty that science and technology
provide and lamenting the cost of those innovations.

Chapter Thirty-Two
A Fly on the Wall

The mathematical patterns that Kepler had found in the heavens looked different from those Galileo had found on Earth. Perhaps that was to be expected. What did falling rocks have to do with endlessly circling planets, which plainly were not falling at all?

Isaac Newton's answer to that question would make use of mathematical tools that Kepler and Galileo did not know. Both astronomers were geniuses, but everything they found might conceivably have been discovered in Greece two thousand years before. To go further would require a breakthrough the Greeks never made.

The insight that eluded Euclid and Archimedes (and Kepler and Galileo as well) supposedly came to René Descartes when he was lying in bed one morning in 1636, idly watching a fly crawl along the wall. (“I sleep ten hours every night,” he once boasted, “and no care ever shortens my slumber.”) The story—so claimed one of Descartes' early biographers—was that Descartes realized that the path the fly traced as it moved could be precisely described in numbers. When the fly first caught Descartes' eye, for instance, it was 10 inches above the floor and 8 inches from the left-hand edge of the wall. A moment later it was 11 inches above the floor and 9 inches from the left edge. All you needed were two lines at right angles—the horizontal line where the wall met the floor, say, and the vertical line from floor to ceiling where two walls met. Then at any moment the fly's position could be pinpointed—this many inches from the horizontal line, that many from the vertical.

Pinpointing a location was an old idea, as old as latitude and longitude. The new twist was to move beyond a static description of the present moment—the fly is 11 inches from here, 9 inches from there; Athens is at 38˚N, 23˚E—and to picture a
moving
point and the path it drew as it moved. Take a circle. It can be thought of in a static way, as a particular collection of points—all those points sitting precisely one inch from a given point, for instance. Descartes pictured circles, and other curves, in a more dynamic way. Think of an angry German Shepherd tethered to a stake and straining to reach the boys teasing him, just beyond his reach. The dog traces a circle—or, more accurately, an arc that forms part of a circle—as he moves back and forth at the end of his taut leash. A six-year-old on a swing, pumping with all his might, traces out part of a circle as the swing arcs down toward the ground and then up again.

From the notion of a curve as a path in time, it was but a step to the graphs that we see every day. The key insight was that the two axes did not necessarily have to show latitude and longitude; they could represent
any
two related quantities. If the horizontal axis depicted “time,” for instance, then a huge variety of numerical changes suddenly took on pictorial form.

The most ordinary graph—changes in housing prices over the last decade, rainfall this year, unemployment rates for the past six months—is an homage to Descartes. A table of numbers might contain the identical information, but a table muffles the patterns and trends that leap from a graph. We have grown so accustomed to graphs that show how something changes as time passes that we forget what a breakthrough they represent. (Countless expressions take this familiarity for granted: “off the charts,” “steep learning curve,” “a drop in the Dow.”) Any run-of-the-mill illustration in a textbook—a graph of a cannonball's position, moment by moment, as it flies through the air, for example—is a sophisticated abstraction. It amounts to a series of stop-action photos. No such photos would exist for centuries after Descartes' death. Only familiarity has dulled the surprise.
⁴¹

Even in its humblest form (in other words, even aside from thinking of a curve as the trajectory of a moving point), Descartes' discovery provided endless riches. With his horizontal and vertical axes in place, he could easily construct a grid—he could, in effect, tape a piece of graph paper to any spot he wanted. That assigned every point in the world a particular address:
x
inches from this axis,
y
inches from that one. Then, for the first time, Descartes could approach geometry in a new way. Rather than think of a circle, say, as a picture, he could treat it as an equation.

A circle consisted of all the points whose
x
's and
y
's combined in a particular way. A straight line was a different equation, a different combination of
x
's and
y
's, and so was every other curve. A curve was an equation; an equation was a curve. This was a huge advance, in the judgment of John Stuart Mill “the greatest single step ever made in the progress of the exact sciences.” Now, suddenly, all the tools of algebra—all the well-developed arsenal of techniques for manipulating equations—could be enlisted to solve problems in geometry.

But it was not simply that algebra could be brought to bear on geometry. That would have been a huge practical breakthrough, but Descartes' insight was a conceptual revolution as well. Algebra and geometry had always been seen as independent subjects. The distinction wasn't subtle. The two fields dealt with different topics, and they looked different. Algebra was a forest of symbols, geometry a collection of pictures. Now Descartes had come along and showed that algebra and geometry were two languages that described a shared reality. This was completely unexpected and hugely powerful, as if today someone suddenly showed that every musical score could be converted into a scene from a movie and every movie scene could be translated into a musical score.

Chapter Thirty-Three
“Euclid Alone Has Looked on Beauty Bare”

Descartes unveiled his new graphs in 1637, in an appendix to a work called
Discourse on Method
. The book is a milestone in the history of philosophy, the source of one of the best known of all philosophical maxims. In the
Discourse
Descartes set out his determination to reject all beliefs that could possibly be incorrect and to build a philosophy founded on indisputable truths. The world and everything in it might be an illusion, Descartes argued, but even if the world was but a dream it was
his
dream, and so he himself could not be merely an illusion. “I think, therefore I am.”

In the same work he added three short afterwords, each meant to demonstrate the power of his approach to philosophy. In an essay called “Geometry,” Descartes talked about curves and moving points; he explained that a curve can be depicted in a picture or captured in an equation and showed how to translate between the two; he discussed graphs and the use of what are known today as Cartesian coordinates. He understood the value of what he had done. “I do not enjoy speaking in praise of myself,” he wrote in a letter to a friend, but he forced himself. His new, graph-based approach to geometry, he went on, represented a leap “as far beyond the treatment in the ordinary geometry as the rhetoric of Cicero is beyond the ABC of children.”

It did. The wonder is that something so useful and so obvious—in hindsight—should have eluded the world's greatest thinkers for thousands of years. But this is an age-old story. In the making of the modern world, the same pattern has recurred time and again: some genius conceives an abstract idea that no one before had ever grasped, and in time it finds its way so deeply into our lives that we forget that it had to be invented in the first place.

Abstraction is always the great hurdle. Alfred North Whitehead argued that it was “a notable advance in the history of thought” when someone hit on the insight that two rocks and two days and two sticks all shared the abstract property of “twoness.” For countless generations no one had seen it.

The same holds for nearly every conceptual breakthrough. The idea that “zero” is a number, for instance, proved even more elusive than the notion of “two” or “seven.” Whitehead again: “The point about zero is that we do not need to use it in the operations of daily life. No one goes out to buy zero fish. It is in a way the most civilized of all the [numbers], and its use is only forced on us by the needs of cultivated modes of thought.” With zero in hand, we suddenly have a tool kit that lets us start building the conceptual world. Zero opens the way to place notation—we can distinguish 23 from 203 from 20,003—and to arithmetic and algebra and countless other spinoffs.

Negative numbers once posed similar mysteries. Today the concept of a $5 bill is easy to understand, and so is a $5 IOU. A temperature of 10 degrees is straightforward, and so is 10 degrees below zero. But in the history of the human race, for the greatest intellects over the course of millennia, the notion of negative numbers seemed as baffling as the idea of time travel does to us. (Descartes wrestled to make sense of how something could be “less than nothing.”) Numbers named amounts—1 goat, 5 fingers, 10 pebbles. What could negative 10 pebbles mean?

(Lest we grow too smug we should remember the dismay of today's students when they meet “imaginary numbers.” The name itself [coined by Descartes, in the same essay in which he explained his new graphs] conveys the unease that surrounded the concept from the start. Small wonder. Students still learn, by rote, that “positive times positive is positive, and negative times negative is positive.” Thus, –2 × –2 = 4, and so is 2 × 2. Then they learn a new definition—an imaginary number is one that, when multiplied by itself, is
negative
! It took centuries and the labors of some of the greatest minds in mathematics to sort it out.)

The ability to conceive strange, unintuitive concepts like “twoness” and “zero fish” and “negative 10 pebbles” lies at the heart of mathematics. Above all else, mathematics is the art of abstraction. It is one thing to see two apples on the ground next to three apples. It is something else to grasp the universal rule that 2 + 3 = 5.

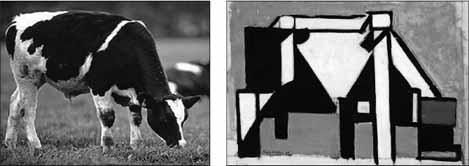
Reality versus abstraction. Photo of cow, left. Painting of cow by Dutch artist Theo van Doesburg, right, © The Museum of Modern Art/licensed by SCALA/Art Resources, NY.

In the history of science, abstraction was crucial. It was abstraction that made it possible to look past the chaos all around us to the order behind it. The surprise in physics, for instance, was that nearly everything was beside the point. Less detail meant more insight. A rock fell in precisely the same way whether the person who dropped it was a beauty in silk or an urchin in rags. Nor did it matter if the rock was a diamond or a chunk of brick, or if it fell yesterday or a hundred years ago, or in Rome or in London.

The skill that physics demanded was the ability to look past particulars to universals. Just as someone working on a geometry problem would not care whether a triangle was drawn in pencil or ink, so a scientist seeking to describe the world would dismiss countless details as true but irrelevant. Much of a modern physicist's early training consists in learning to transform colorful questions about such things as elephants tumbling down mountainsides into abstract diagrams showing arrows and angles and masses.

The move from elephants to ten-thousand-pound masses echoes the transformation from Aristotle's worldview to Galileo's. The battle between the two approaches was as sweeping as a contest can be, far more than a debate over whether the sun circled the Earth or vice versa, big as that issue was. The broader questions had to do with how to study the physical world. For Aristotle and his followers, the point of science was to engage with the real world in all its complexity. To talk of weights plummeting through vacuums or perfect spheres rolling forever across infinite planes was to mistake idealized diagrams for reality. But the map was not the territory. Explorers needed to grapple with the world as it is, not with a dessicated and lifeless counterpart.

In Galileo's view, this was exactly backward. The way to understand the world was not to focus on its every quirk and blemish but to look beyond those distractions to the deeper truths they obscured. When Galileo talked about whether heavy objects fall faster than light ones, for instance, he imagined ideal circumstances—objects falling in a vacuum rather than through the air—in order to avoid the complications posed by air resistance. But Aristotle insisted that no such thing as a vacuum could exist in nature (it was impossible, because objects fall faster in a thin medium, like water, than they do in a thick one, like syrup. If there were vacuums, then objects would fall infinitely fast, which is to say they would be in two places at once).
⁴²
Even if a vacuum could somehow be contrived, why would anyone think that the behavior of objects in those peculiar conditions bore any relation to ordinary life? To speculate about what might happen in unreal circumstances was an exercise in absurdity, like debating whether ghosts can get sunburns.

Galileo vehemently disagreed. Abstraction was not a distortion but a means of seeing truth unadorned. “Only by imagining an impossible situation can a clear and simple law of fall be formulated,” in the words of the late historian A. Rupert Hall, “and only by possessing that law is it possible to comprehend the complex things that actually happen.”

By way of explaining what the abstract, idealized world of mathematics has to do with the real world, Galileo made an analogy to a shopkeeper measuring and weighing his goods. “Just as the accountant who wants his calculations to deal with sugar, silk, and wool must discount the boxes, bales and other packings, so the mathematical scientist . . . must deduct the material hindrances” that might entangle him.

The importance of abstraction was a crucial theme, and Galileo came back to it often. At one point he exchanged his shopkeeper image for a more poetic one. With abstraction's aid, he wrote, “facts which at first sight seem improbable will . . . drop the cloak which has hidden them and stand forth in naked and simple beauty.”

Galileo won his argument, and science has never turned back. Mathematics remains the language of science because, ever since Galileo, we have taken for granted that abstraction is the pathway to truth.