The Clockwork Universe (27 page)

Chapter Forty-Five
The Apple and the Moon

The greatest scientific triumph of the seventeenth century, Newton's theory of universal gravitation, was in a sense a vehicle for showing off the power and range of the mathematical techniques that Newton and Leibniz had fought to claim. Both men discovered calculus, but it was Newton who provided a stunning demonstration of what it could do.

Until 1687, Isaac Newton had been known mainly, to those who knew him at all, as a brilliant mathematician who worked in self-imposed isolation. No recluse ever broke his silence more audaciously.

Fame came with the publication of the
Principia
. Newton had been at Cambridge for two decades. University rules required that he teach a class or two, but this did not impose much of a burden, either on Newton or anyone else. “So few went to hear Him, & fewer that understood him,” one contemporary noted,
“that oftimes he did in a manner, for want of Hearers, read to
ye Walls.”

As Newton told the story, his rise to fame had indeed begun with the fall of an apple. In his old age he occasionally looked back on his career, and eager listeners noted down every word. A worshipful young man named John Conduitt, the husband of Newton's niece, was one of several who heard the apple story firsthand. “In the year 1666 he retired again from Cambridge . . . to his mother in Lincolnshire,” Conduitt wrote, “& whilst he was musing in a garden it came into his thought that the power of gravity (which brought an apple from the tree to the ground) was not limited to a certain distance from the earth but that this power must extend much farther than was usually thought. Why not as high as the moon said he to himself & if so that must influence her motion & perhaps retain her in her orbit, whereupon he fell a calculating. . . .”

The story, which is the one thing everyone knows about Isaac Newton, may well be a myth.
⁴⁸
Despite his craving for privacy, Newton was acutely aware of his own legend, and he was not above adding a bit of gloss here and there. Historians who have scrutinized his private papers believe that his understanding of gravity dawned slowly, over several years, rather than in a flash of insight. He threw in the apple, some suspect, simply for color.

In any case, it wouldn't have taken an apple to remind Newton that objects fall. Everyone had always known that. The point was to look beyond that fact to the questions it raised. If apples fell to the ground because some force drew them, did that force extend from the tree's branches to its top? And beyond the top to . . . to where? To the top of a mountain? To the clouds? To the moon? Those questions had seldom been asked. There were many more. What about the apple when it was
not
falling? An apple in a tree stays put because it is attached to the branch. No surprise there. But what about the moon? What holds the moon up in the sky?

Before Newton the answer had two parts. The moon stayed in the sky because that was its natural home and because it was made of an ethereal substance that was nothing like the heavy stuffing of bodies here on Earth. But that would no longer do. If the moon was just a big rock, as telescopes seemed to show, why didn't it fall like other rocks?

The answer, Newton came to see, was that
it does
fall
. The breakthrough was to see how that could be. How could something fall and fall but never arrive? Newton's answer in the case of the moon, a natural satellite, ran much like the argument we have already seen, for an artificial satellite.

We tend to forget the audacity of that explanation, and Newton's plain tone helps us along in our mistake. “I began to think of gravity extending to ye orb of the Moon,” he recalled, as if nothing could have been more natural. Newton began to give serious thought, in other words, to asking whether the same force that pulled an apple to the Earth also pulled the moon toward the Earth. But this is to downplay two feats of intellectual daring. Why should anyone have thought that the moon is falling, first of all, when it is plainly hanging placidly in the sky, far beyond our reach or the reach of anything else? And even if we did make the large concession that it is falling, second of all, why should that fall have anything in common with an apple's fall? Why would anyone presume that the same rules governed realms as different as heaven and Earth?

But that is exactly what Newton did presume, for aesthetic and philosophical reasons as much as for scientific ones. Throughout his life Newton believed that God operated in the simplest, neatest, most efficient way imaginable. That principle served as his starting point whether he was studying the Bible or the natural world. (We have already noted his insistence that “it is ye perfection of God's works that they are all done with ye greatest simplicity.”) The universe had no superfluous parts or forces for exactly the reason that a clock had no superfluous wheels or springs. And so, when Newton's thoughts turned to gravity, it was all but inevitable that he would wonder how much that single force could explain.

Newton's first task was to find a way to turn his intuition about the sweep and simplicity of nature's laws into a specific, testable prediction. Gravity certainly seemed to operate here on Earth; if it did reach all the way to the moon, how would you know it? How would gravity reveal itself? To start with, it seemed clear that if gravity did extend to the moon, its force must diminish over that vast distance. But how much? Newton had two paths to an answer. Fortunately, both gave the same result.

First, he could try intuition and analogy. If we see a bright light ten yards off, say, how bright will it be if we move it twice as far away, to twenty yards distance? The answer was well-known. Move a light twice as far away and it will not be half as bright, as you might guess, but only one-fourth as bright. Move it ten times as far away and it will be one-hundredth as bright. (The reason has to do with the way light spreads. Sound works the same way. A piano twenty yards away sounds only one-fourth as loud as a piano ten yards away.)

So Newton might have been tempted to guess that the pull of gravity decreases with distance in the same way that the brightness of light does. Physicists today talk about “inverse-square laws,” by which they mean that some forces weaken not just in proportion to distance but in proportion to distance squared. (It would later turn out that electricity and magnetism follow inverse-square laws, too.)

A second way of looking at gravity's pull gave the same answer. By combining Kepler's third law, which had to do with the size and speed of the planets' orbits, with an observation of his own about objects traveling in a circle, Newton calculated the strength of gravity's pull. Again, he found that gravity obeyed an inverse-square law.

Now came the test. If gravity actually pulled on the moon, how much did it pull? Newton set to work. He knew that the moon orbits the Earth. It travels in a circle, in other words, and not in a straight line. (To be strictly accurate, it travels in an ellipse that is almost but not quite circular, but the distinction does not come into play here.) He knew, as well, what generations of students have since had drummed into them as “Newton's first law”—in modern terms,
a body in motion will travel in a straight line at a steady speed unless some force acts on it (and a body at rest will stay at rest unless some force acts on it).

So some force was acting on the moon, pulling it off a straight-line course. How far off course? That was easy to calculate. To start with, Newton knew the size of the moon's orbit, and he knew that the moon took a month to travel once around that circuit. Taken together, those facts told him the moon's speed. Next came a thought experiment. What would happen to the moon if gravity were magically turned off for a second? Newton's first law gave him the answer—it would shoot off into space on a straight line, literally going off on a tangent. (If you tied a rock with a piece of string and swung it around your head, the rock would travel in a circle until the string snapped, and then it would fly off in a straight line.)

But the moon stays in its circular orbit. Newton knew what that meant. It meant a force was pulling it. Now he needed some
numbers. To find out how far the moon was pulled, all he had to
do was calculate the distance between where the moon actually is
and where it would have been if it had traveled in a straight line. That distance was the fall Newton was looking for—the moon
“falls” from a hypothetical straight line to its actual position.

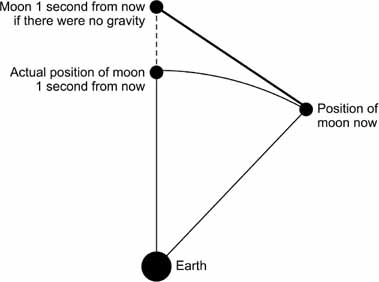
Newton calculated the distance the moon falls in 1 second, which corresponds to the dashed line in the diagram.

In his quest to compare Earth's pull on the moon and on an apple, Newton was nearly home. He knew how far the moon falls in one second. He had just calculated that. It falls about
1
/
20
of an inch. He knew how far an apple falls in one second. Galileo had found that out, with his ramps: 16 feet.

All that remained was to look at the ratio of those two falls, the ratio of
1
/
20
of an inch to 16 feet. The last puzzle piece was the distance from the Earth to the moon. Why did that matter? Because the distance from the Earth to the moon was about 60 times the distance from the center of the Earth to the Earth's surface. Which was to say that the moon was 60 times as far from the center of the Earth as the apple was. If gravity truly did follow an inverse-square law, then the Earth's pull on the moon should be 3,600 times weaker (60 × 60) than its pull on the apple.

Only the last, crucial calculation remained. The moon fell
1
/
20
of an inch in one second; an apple fell 16 feet in one second.
Was the ratio of
1
/
20
of an inch to 16 feet the same as the ratio
of 1 to 3,600, as Newton had predicted? How did the moon's fall compare with the apple's fall?

Just as Newton had hoped it would, or nearly so. The two ratios almost matched. Newton “compared the force required to keep the Moon in her Orb with the force of gravity,” he wrote proudly, “& found them answer pretty nearly.” The same calculation carried out today, with far better data than Newton had available, would give even closer agreement. That wasn't necessary. The big message was already clear. Gravity reached from the Earth to the moon. The same force that drew an apple drew the moon. The same law held here and in the heavens. God had indeed designed his cosmos with “ye greatest simplicity.”

Chapter Forty-Six
A Visit to Cambridge

Newton's moon calculation had buttressed his faith in simple laws, but he still had an immense distance to cover before he could prove his case. The moon was not the universe. What of Kepler's laws, for instance? The great astronomer had devoted his life to proving that the planets traveled around the sun in ellipses. How did ellipses fit in God's cosmic architecture?

Stymied by the difficulty of sorting out gravity, or perhaps
tempted more by questions in other fields, Newton had put gravity
aside after his miracle years. He had made his apple-and-moon calculation when he was in his twenties. For the next twenty years he
gave most of his attention to optics, alchemy, and theology instead.

Late on a January afternoon in 1684, Robert Hooke, Christopher Wren, and Edmond Halley left a meeting of the Royal Society and wandered into a coffeehouse to pick up a conversation they had been carrying on all day. Coffee had reached England only a generation before, but coffeehouses had spread everywhere.
⁴⁹
Hooke in particular seemed to thrive in the rowdy atmosphere. In crowded rooms thick with the hubbub of voices and the smells of coffee, chocolate, and tobacco, men sat for hours debating business, politics, and, lately, science. (Rumors and “false news” spread so quickly, as with the Internet today, that the king tried, unsuccessfully, to shut coffeehouses down.)

With steaming mugs in hand, the three men resumed talking of astronomy. All three had already guessed, or convinced themselves by the same argument Newton had made using Kepler's third law, that gravity obeyed an inverse-square law. Now they wanted the answer to a related question—if the planets did follow an inverse-square law, what did that tell you about their orbits? This question—in effect,
where do Kepler's laws come from?—
was one of the central riddles confronting all the era's scientists.

Halley, a skilled mathematician, admitted to his companions that he had tried to find an answer and failed. Wren, still more skilled, confessed that
his
failures had stretched over the course of several years. Hooke, who was sometimes derided as the “universal claimant” for his habit of insisting that every new idea that came along had occurred to him long before, said that he'd solved this problem, too. For the time being, he said coyly, he preferred to keep the answer to himself. “Mr. Hook said that he had it,” Halley recalled later, “but that he would conceale it for some time, that others trying and failing might know how to value it, when he should make it publick.”

Wren, dubious, offered a forty-shilling prize—roughly four hundred dollars today—to anyone who could find an answer within two months. No one did. In August 1684, Halley took the question to Isaac Newton. Halley, one of the few great men of the Royal Society who was charming as well as brilliant, scarcely knew Newton, though he knew his mathematical reputation. But Halley could get along with everyone, and he made a perfect ambassador. Though still only twenty-eight, he had already made his mark in mathematics and astronomy. Just as important, he was game for anything. In years to come he would stumble through London's taverns with Peter the Great, on the czar's visit to London; he would invent a diving bell (in the hope of salvaging treasure from shipwrecks) and would descend deep underwater to test it himself; he would tramp up and down mountains to compare the barometric pressure at the summit and the base; in an era of wooden ships he would survey vast swaths of the world's oceans, from the tropics to “islands of ice.”

Now his task was to win over Isaac Newton. “After they had been some time together,” as Newton later told the story to a colleague, Halley explained the reason for his visit. He needed Newton's help. The young astronomer spelled out the question that had stumped him, Wren, and Hooke. If the sun attracted the planets with a force that obeyed an inverse-square law, what shape would the planets' orbits be?

“Sir Isaac replied immediately that it would be an Ellipsis.” Halley was astonished. “The Doctor struck with joy & amaze
ment asked him how he knew it. Why saith he I have calculated it.”

Halley asked if he could see the calculation. Newton rummaged through his papers. Lost. Halley extracted a promise from Newton to work through the mathematics again, and to send him the results.