Warped Passages (36 page)

Zooming In and Out
Effective field theories
apply the effective theory idea that we learned about in Chapter 1 to quantum field theory. They focus only on those energy and length scales you can hope to measure. The effective field theory that applies at a particular energy or distance scale “effectively” describes those energies or distances we need to take into account. It concentrates on those forces and interactions that can occur when particles have that particular energy (or lower)
*
and ignores any energies that are inaccessibly higher. It doesn’t ask for the details of physical processes or particles that occur only with higher energy than you can achieve.
One advantage of an effective field theory is that even if you don’t know what interactions take place over short distances, you can still study the quantities that matter at the scales that interest you. You really need only to think about the quantities that you can (in principle) detect. When you mix paint, you don’t need to know its detailed molecular structure. But you probably want to know the properties that you readily perceive, like color and texture. With this information, even without knowing the microstructure of your paint, you could categorize the paints’ relevant properties and predict what mixtures of the paints would look like when you applied them to your canvas.
However, if you did know your paint’s chemical composition, the rules of physics would allow you to deduce some of those properties. You don’t need this information when you’re painting (using the effective theory) but you would find it useful if you were making paint (deriving the effective theory’s parameters from a more fundamental theory).
Similarly, if you don’t know the short-distance (high-energy) theory, you won’t be able to derive measurable quantities. However, when you do know the short-distance details, quantum field theory tells you
precisely how to relate the different effective theories that apply to different energies. It lets you derive the quantities of one effective theory, such as masses or interaction strength, from the quantities of another.
The method for calculating how quantities depend on energy or distance, which was first developed by Kenneth Wilson in 1974, has a fancy name: the
renormalization group
. Along with symmetries, two of the most powerful tools in physics are the effective theory concept and the renormalization group, both of which involve physical processes with very different lengths or energy scales. The word “group” is a mathematical term that stuck, although its mathematical origin is largely irrelevant.
Renormalization is not such a bad word, though. It refers to the fact that at each distance scale of interest, you pause to get your bearings. You determine which particles and which interactions are relevant at the particular energies that interest you at the moment. You then apply a new normalization—that is, a new calibration—for any parameters in the theory.
The renormalization group uses ideas that are similar to those set out in Chapter 2, where we discussed the feasibility of interpreting a higher-dimensional theory in lower-dimensional language and treated a two-dimensional theory that had a small rolled-up dimension as if it were only one-dimensional. When we curled up dimensions, we ignored all the details of what happened inside the extra dimensions and assumed that everything could be described in lower-dimensional terms. Our new “normalization” was the four-dimensional description that could be used when focusing on large distances.
We can use a very similar procedure to derive a theory that applies to long distances from any theory appropriate to short distances: decide the minimum length you care about, and “wash out” the physics relevant to shorter scales. One way of doing this is to find the average value of those quantities whose details would make a difference only at the shorter distances you have chosen to ignore. If you had a grid filled with grayscale dots, you would literally average the shade density of the smaller dots to find the shade for bigger dots that would reproduce their effect. Your eyes do this automatically when you view something with fuzzy resolution.
If you can see things only with a given level of precision, you don’t need to know what happens on smaller scales to make useful calculations that relate measurable quantities. Your most efficient course often involves choosing the “pixel size” in your theory to agree with your level of precision. That way, you can neglect heavy particles that you’ll never produce and short-distance interactions that will never occur. Instead, you can focus your calculations on particles and interactions that are relevant at the energy you can achieve.
However, if you do know the more precise theory that applies at smaller distances, you can use that information to calculate quantities in the effective theory that interests you—that is, the effective theory with lower resolution. Just as with the grayscale dots, when you go from an effective theory with short-distance resolution to another with less precise resolution, in essence you change the “pixel size” with which you choose to analyze your theory. The renormalization group tells you how to calculate the influence that such short-distance interactions could have on the particles in your long-distance theory. You extrapolate physical processes from one length or energy scale to another.
Virtual Particles
Renormalization group calculations make these extrapolations by taking into account the effect of quantum mechanical processes and
virtual particles
. Virtual particles, a consequence of quantum mechanics, are strange, ghostly twins of actual particles. They pop in and out of existence, lasting only the barest moment. Virtual particles have the same interactions and the same charges as physical particles, but they have energies that look wrong. For example, a particle moving very fast clearly carries a lot of energy. A virtual particle, on the other hand, can have enormous speed but no energy. In fact, virtual particles can have any energy that is different from the energy carried by the corresponding true physical particle. If it had the same energy, it would be a real particle, not a virtual one. Virtual particles are a strange feature of quantum field theory that you have to include to make the right predictions.
So how can these apparently impossible particles exist? A virtual particle with its borrowed energy could not exist were it not for the uncertainty principle, which allows particles to have the wrong energy so long as they do so for such a short time that it would never be measured.
The uncertainty principle tells us that it would take infinitely long to measure energy (or mass) with infinite precision, and that the longer a particle lasts, the more accurate our measurement of its energy can be. But if the particle is short-lived and its energy cannot possibly be determined with infinite precision, the energy can temporarily deviate from that of a true long-lived particle. In fact, because of the uncertainty principle, particles will do whatever they can get away with for as long as they can. Virtual particles have no scruples and misbehave whenever no one is watching. (A physicist from Amsterdam even suggested that they are Dutch.)
You can think of the vacuum as a reservoir of energy—virtual particles are particles that emerge from the vacuum, temporarily borrowing some of its energy. They exist only fleetingly and then disappear back into the vacuum, taking with them the energy they borrowed. That energy might return to its place of origin, or it might be transferred to particles in some other location.
The quantum mechanical vacuum is a busy place. Even though the vacuum is by definition empty, quantum effects give rise to a teeming sea of virtual particles and antiparticles that appear and disappear—even though no stable, long-lasting particles are present. All particle-antiparticle pairs can in principle be produced, albeit only for very short visits, too short to be seen directly. But however brief their existence, we care about virtual particles because they nonetheless leave their imprint on the interactions of long-lived particles.
Virtual particles have measurable consequences because they influence the interactions of the real physical particles that enter and leave an interaction region. During its brief span of its existence, a virtual particle can travel between real particles before disappearing and repaying its energy debt to the vacuum. Virtual particles thereby act as intermediaries that influence the interactions of long-lived stable particles.
For example, the photon in Figure 47 (Chapter 7), which was
exchanged to generate the classical electromagnetic force, was in fact a virtual photon. It didn’t have the energy of a true photon, but it didn’t have to. It only needed to last long enough to communicate the electromagnetic force and make the real charged particles interact.
Another example of virtual particles is shown in Figure 59. Here, a photon enters an interaction region, a virtual electron-positron pair is produced, and then the pair is absorbed at another location. At the place where the particles are absorbed, another photon emerges from the vacuum that carries off the energy that the intermediate electron-positron pair temporarily borrowed. We’ll now investigate one remarkable consequence of this type of interaction.
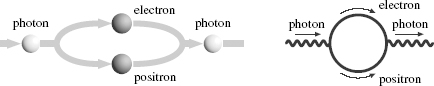
Figure 59.
A real physical photon can turn into a virtual electron and a virtual positron, which can then turn back into a photon. This is illustrated with a Feynman diagram on the right and schematically on the left.
Why Interaction Strength Depends on Distance
The strengths of the forces we know about depend on the energies and distances involved in particle interactions, and virtual particles play a part in that dependence. For example, the strength of the electromagnetic force is smaller when two electrons are further apart. (Remember, this quantum mechanical decrease is over and above the classical distance dependence of electromagnetism.) The consequences of virtual particles and the distance dependence of forces is real; theoretical predictions and experiments match extremely well.
The reason that the quantities of an effective theory—the strength of forces or interactions, for example—depend on the energies and the separations of the particles involved follows from a feature of quantum field theory that the physicist Jonathan Flynn jokingly called
the
anarchic principle
.
*
The anarchic principle follows from quantum mechanics, which tells us that all particle interactions that can happen will happen. In quantum field theory, everything that is not forbidden will occur.
I’ll call each separate process by which a particular group of physical particles interacts a
path
. A path may or may not involve virtual particles. When it does, I’ll call that path a
quantum contribution
. Quantum mechanics tells us that all possible paths contribute to the net strength of an interaction. For example, physical particles can turn into virtual particles, which can interact with each other and then turn back into other physical particles. In such a process, the original physical particles might reemerge or they might turn into different physical particles. Even though the virtual particles wouldn’t last long enough for us to observe them directly, they would nonetheless affect the way real observable particles interacted with one another.
Trying to prevent virtual particles from facilitating an interaction would be like telling certain of your friends a secret and hoping it won’t reach another friend. You know that sooner or later, some of the “intermediate virtual” friends will betray your confidence and relay the message to that other friend. Even if you already told that friend your secret, the fact that your virtual friends will discuss it with him as well will affect his opinion on the subject, too. In fact, his opinion will be the net result of everyone he has talked to.
Not only direct interactions between physical particles, but also
indirect interactions
—those that involve virtual particles—play a role in communicating forces. Just as your friend’s opinion is affected by everyone who talks to him, the net interaction between particles is the sum of all possible contributions, including those from virtual particles. And because the importance of virtual particles depends on the distances involved, the strengths of forces depend on distance.
The renormalization group tells us precisely how to calculate the
impact of virtual particles in any interaction. All of the effects of intermediate virtual particles are added together, and this either strengthens or impedes the strength of a gauge boson’s interactions.
Indirect interactions play a more important role when interacting particles are further apart. A greater distance is analogous to telling your secret to more “virtual” friends. Although you can’t be sure that any single friend will betray your confidence, the more friends you tell, the more likely it is that at least one of them will. Whenever a path exists by which virtual particles can contribute to the net strength of an interaction, quantum mechanics ensures that they will. And the amount by which virtual particles affect that strength depends on the distance over which the force is communicated.