Warped Passages (40 page)

The other energy is the Planck scale energy, which is sixteen orders of magnitude, or ten million billion times, greater than the weak scale energy: a whopping 10
19
GeV. The Planck scale energy determines the strength of gravitational interactions: Newton’s law says that the strength is
inversely
proportional to the second power of that energy. And because the strength of gravity is small, the Planck scale mass (related to the Planck scale energy by
E
=
mc
2
) is big. A huge Planck scale mass is equivalent to extremely feeble gravity.
So far, the Planck scale mass hasn’t come up in our particle physics
discussions because gravity is so weak that for most particle physics calculations it can safely be ignored. But that is precisely the question particle physicists want answered: why is gravity so weak that it can be ignored in particle physics calculations? Another way of phrasing the hierarchy problem is to ask why the Planck scale mass is so huge—why is it ten million billion times higher than the masses relevant to particle physics scales, all of which are less than a few hundred GeV?
To give you a basis for comparison, consider the gravitational attraction between two low-mass particles, such as a pair of electrons. This gravitational attraction is about a hundred million trillion trillion trillion times weaker than the electric repulsion between the electron pair. The two kinds of forces would be comparable only if electrons were heavier than they really are by a factor of ten billion trillion. That’s an enormous number—it’s comparable to the number of times you could lay the island of Manhattan end to end in the extent of the observable universe.
The Planck scale mass is enormously bigger than the electron’s mass and all other particle masses we know of, and that signifies that gravity is very much weaker than the other known forces. But why should there be such a huge discrepancy between the strengths of most forces—or, equivalently, why should the Planck scale mass be so enormous compared with known particle masses?
To particle physicists, the enormous ratio between the Planck scale mass and the weak scale mass, a factor of about ten million billion, is hard to stomach. This ratio is greater than the number of minutes that have passed since the Big Bang; it’s about a thousand times the number of marbles you can line up from the Earth to the Sun. It’s more than a hundred times the number of pennies in the U.S. budget deficit! Why should two masses that describe the same physical system be so enormously different?
If you are not a particle physicist, this might not sound like a very significant problem in itself, even though those numbers are dramatically big. After all, we can’t necessarily explain everything, and two masses just might be different for no very good reason. But the situation is actually far worse than it appears. Not only is there the unexplained enormous mass ratio. In the following section, we’ll
see that in quantum field theory, any particle that interacts with the Higgs particle can participate in a virtual process that raises the Higgs particle mass to a value as high as the Planck scale mass, 10
19
GeV.
In fact, if you asked any honest particle physicist who knew gravity’s strength but knew nothing about the measured weak gauge boson masses to estimate the Higgs particle’s mass using quantum field theory, he would predict a value for the Higgs particle—and hence the weak gauge boson masses—that is ten million billion times too large. That is, he would conclude from his calculation that the ratio between the Planck scale mass and the mass of the Higgs particle (or the weak scale mass, which is determined by the Higgs particle’s mass) should be far closer to unity than to ten million billion! His estimate of the weak scale mass would be so close to the Planck scale mass that particles would all be black holes, and particle physics as we know it would not exist. Although he would have no a priori expectation for the value of either the weak scale mass or the Planck scale mass individually, he could use quantum field theory to estimate the ratio—and he would be totally wrong. Clearly, there is an enormous discrepancy here. The next section explains why.
Virtual Energetic Particles
The reason that the Planck scale mass enters quantum field theory calculations is a subtle one. As we have seen, the Planck scale mass determines the strength of the gravitational force. According to Newton’s law, the gravitational force is inversely proportional to the value of the Planck scale mass, and the fact that gravity is so weak tells us that the Planck scale mass is huge.
Generally, we can ignore gravity when making predictions in particle physics because the gravitational effects on a particle with mass of about 250 GeV are completely negligible. If you really need to account for gravitational effects you can systematically incorporate them, but it’s not usually worth the bother. Later chapters will explain the new and very different scenarios in which higher-dimensional gravity is strong and cannot be neglected. But for the conventional,
four-dimensional Standard Model, neglecting gravity is a standard and justifiable practice.
However, the Planck scale mass has another role as well: it is the maximum mass that virtual particles can take in a reliable quantum field theory calculation. If particles carried more mass than the Planck scale mass, the calculation would be untrustworthy, and general relativity would not be reliable and would have to be replaced by a more comprehensive theory, such as string theory.
But when particles (including virtual particles) have mass that is less than the Planck scale mass, conventional quantum field theory should apply and calculations based on quantum field theory should be trustworthy. That means that a calculation involving a virtual top quark (or any other virtual particle) with mass almost as big as the Planck scale mass should be reliable.
The problem for the hierarchy is that the contribution to the Higgs particle’s mass from virtual particles with extremely high mass will be about as big as the Planck scale mass, which is ten million billion times greater than the Higgs particle mass we want—the one that will give the right weak scale mass and elementary particle masses.
If we consider a path, such as the one shown in Figure 62, in which the Higgs particle turns into a virtual top quark-antitop quark pair, we can see that the contribution to the Higgs particle’s mass will be far too large. In fact, any type of particle that can interact with the Higgs particle might appear as a virtual particle and have mass
*
up
to the Planck scale mass. And the result of all these possible paths is huge quantum contributions to the Higgs particle’s mass. The Higgs particle has to be much less massive.
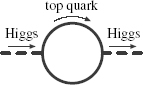
Figure 62.
A contribution to the Higgs particle’s mass from a virtual top quark and a virtual antitop quark. The Higgs particle can convert to a virtual top quark and virtual antitop quark, and this gives an enormous contribution to the Higgs particle’s mass.
Particle physics in its present state is like a too effective “trickle-down” theory. In economics, a hierarchy of wealth is not difficult to achieve. The application of trickle-down economics has never raised poor people’s financial well-being much at all, let alone to the level of the upper classes. In physics, though, the transfer of wealth is far too efficient. If one mass is large, then quantum contributions tell us that all masses of elementary particles are expected to be about as large. All particles end up rich in mass. But we know from measurements that high mass (the Planck scale mass) and low mass (particle masses) coexist in our world.
Without modifying or extending the Standard Model, particle physics theory can achieve a small mass for the Higgs particle only through a miraculous value for its classical mass. That value must be extremely large—and possibly negative—so that it can precisely cancel the large quantum contributions. All the mass contributions must add up to 250 GeV.
For this to happen, as in the Grand Unification Theory we considered earlier, the mass must be a fine-tuned parameter. And this fine-tuned parameter would have to be an enormous yet amazingly exact fudge specifically designed to give a small net mass to the Higgs particle. Either the quantum contributions from virtual particles or the classical contribution must be negative, and almost equal in magnitude to the other. The positive and negative terms, each of which is sixteen orders of magnitude too large, must add up to a much smaller value. The required fine-tuning, which must have sixteen-digit accuracy, is more extreme than the fine-tuning required to make your pencil stand on end. It’s about as likely as someone randomly winning the guessing game with Ike.
Particle physicists would prefer a model that didn’t involve the fine-tuning that is required in the Standard Model to ensure a light Higgs particle. Although we might fine-tune in an act of desperation, we hate it. Fine-tuning is almost certainly a badge of shame reflecting our ignorance. Unlikely things sometimes happen, but rarely when you want them to.
The hierarchy problem is the most urgent of the mysteries confronting the Standard Model. To put a positive spin on things, the hierarchy problem provides a clue to what plays the role of the Higgs particle and breaks the electroweak symmetry.
Any theory that replaces the two-field Higgs theory should naturally accommodate or predict a low electroweak mass scale—otherwise it is just not worth thinking about. Many underlying theories are compatible with the physical phenomena we see, but very few of them address the hierarchy problem and include a light Higgs particle in a compelling manner that avoids fine-tuning. While the task of unifying forces is a fascinating, if potentially tenuous, theoretical lure from high-energy physics, the task of solving the hierarchy is a concrete necessity urging progress at relatively low energies. What makes this challenge most exciting is that anything that addresses the hierarchy problem should have experimental consequences that will be measurable at the Large Hadron Collider, where experimenters expect to find particles with masses of about 250 to 1,000 GeV. Without such additional particles, there is no way to get around the problem. We’ll soon see that the experimental consequences of solving the hierarchy problem might be the supersymmetric partners or the particles that travel in extra dimensions that we’ll discuss later on.
What to Remember
- Although we know that the Higgs mechanism is responsible for particle masses, the simplest known example that implements the Higgs mechanism works only with a huge fudge. In the simplest theory you’d expect the masses of weak gauge bosons and quarks to be about ten million billion times greater than they are. The
hierarchy problem
is the question of why this is not the case. - The hierarchy problem arises from the discrepancy between the low weak scale mass and the enormous Planck scale mass (see Figure 63). This latter mass is important for gravity—the large value of the Planck scale mass tells us that gravity is very weak. So another way of phrasing the hierarchy problem is to ask why gravity is so feeble, so much weaker than the other nongravitational forces.
- Any theory that solves the hierarchy problem will be experimentally testable because it will necessarily have experimental implications at colliders operating at energies above the weak scale energy. The Large Hadron Collider will explore such energies very soon.
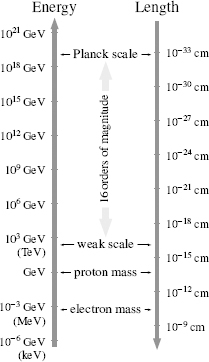
Figure 63.
The hierarchy problem is the question of why the Planck scale energy is so much bigger than the weak scale energy.
Supersymmetry: A Leap Beyond the Standard Model
You were meant for me.
And I was meant for you.
Gene Kelly (“Singing in the Rain”)
When Icarus first arrived in Heaven, he was directed to an orientation seminar where the authorities explained the local rules. To his surprise, he learned that right-wing religious groups were essentially correct, and family values were indeed a cornerstone of his new environment. The authorities had long ago established a traditional family structure premised on the separation of generations and the stability of marriages; a top would always marry a bottom, a charmer would always align with a strange bird, and an uptown girl would always marry a downtown cool cat. Everyone, including Ike, was satisfied with the arrangement.