What is Life?:How chemistry becomes biology (9 page)

All living things involve chemical reactions, thousands of them, and the living cell, the basic unit comprising all life, is a highly complex set of these reactions somehow integrated into a coordinated whole. This fact alone makes the problem of understanding the living state of matter and the elucidation of its underlying characteristics a difficult one. How can that complex interplay of reactions and the molecular entities on which they operate be unravelled? Are some reactions central while others are peripheral? Of course, if we are seeking a better understanding of the reactions of life, we first need to understand chemical reactions in general. What is a chemical reaction and why do they take place? So let us begin by making some general comments about chemical reactivity. The subject is complex, one that requires textbook coverage for a proper treatment. Here I will give a greatly simplified version that primarily addresses those aspects of reactivity that we will need for our
subsequent analysis. Our analysis will reveal that there
is
something very special within the set of chemical reactions that constitute life and understanding what that special feature is will be a focus of the ensuing chapters.

All chemical reactions involve the transformation of some chemical material into some other material. The neutralization of an acid by a base, the degradation of a protein into its constituent amino acid building blocks, the explosive reaction of a mixture of hydrogen and oxygen gases to give water, are all examples of common chemical reactions. This last reaction, that of hydrogen and oxygen gases, occurs very readily—a spark or the presence of a catalyst (for example, metallic platinum or palladium) is all that is needed for it to take place. The reverse reaction in which water spontaneously breaks up into hydrogen and oxygen gases does not occur. Why is that? What governs the direction of a chemical reaction? Broadly speaking, the answer is given by a central law of chemistry, one we have already met briefly—the Second Law of Thermodynamics.

The Second Law is actually a fundamental law of physics, so its wide applicability means that it has a number of different formulations. But in the present context it will suffice to say that chemical reactions proceed such that
less stable
materials are transformed into
more stable
materials. A ball rolling down a slope is a useful analogy. Chemical reactions proceed in a ‘downhill direction’, where downhill signifies toward more stable products, products that are characterized by what is termed lower ‘free energy’. Since the free energy of water is lower than the free energy of a mixture of hydrogen and oxygen gases, the two gases react to form water, and the energy that was stored in the higher-energy hydrogen and oxygen molecules is released as heat. The reverse reaction in which water would be
transformed into hydrogen and oxygen gases cannot take place spontaneously because that would be equivalent to a ball rolling uphill.

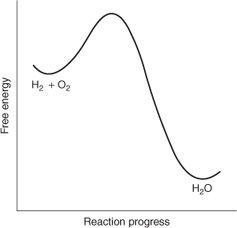
Fig. 2.
Diagram illustrating the free energy change for the reaction of hydrogen and oxygen gases (H
₂
+ O
₂
) to give water (H
₂
O).

The relative free energies of a hydrogen and oxygen mixture compared with that of water are shown schematically in Fig. 2. The hydrogen and oxygen molecules on the left side of the diagram (H
₂
+O
₂
) are located at higher energy than the water product (H
₂
O) on the right side of the diagram.

The diagram also reveals another important point—the hydrogen and oxygen reactants are separated from the water product by a barrier. Even though the hydrogen and oxygen gas mixture is higher in free energy than water, the path leading from reactants to products does not go downhill smoothly. It climbs uphill to some extent before it begins to descend, which means that before the reaction can proceed, the barrier must first be overcome. That’s why a spark
or catalyst is needed to get the reaction going. The spark provides the initial energy boost in order to get the reactants over the barrier, after which the downhill trajectory of the reaction profile takes care of the rest. A catalyst may obviate the need for a spark by reducing the barrier height so that no activation is needed and the reaction can proceed without that energy boost.

Two important lessons can be learnt from the above example. First, reactions will only take place if the reaction products are of lower free energy than the reactants. That determines the direction of any chemical reaction and is called the thermodynamic consideration. Accordingly, the Second Law of Thermodynamics indicates beforehand which reactions are possible and which are not. Once a reaction mixture has reached the lowest possible free energy state for that particular combination of materials, the system is said to be at equilibrium and no further reaction will take place. Like balls at the bottom of a valley, they have nowhere lower to roll. But the fact that a reaction mixture is not at equilibrium, i.e., not in that lowest possible free energy state, does not mean it will necessarily react. If that reaction system is trapped in a local minimum, that is, behind a barrier, it may not be able to overcome the barrier that separates that local minimum from the deeper, product minimum, much like a ball that is trapped in a hollow halfway down some slope. That’s why hydrogen and oxygen gases may be mixed without any reaction taking place if neither catalyst nor spark are provided. These simple notions can now be expressed in the language of chemistry: a reaction that is allowed thermodynamically may or may not proceed, depending on kinetic factors (the barrier height). However, a reaction that is forbidden thermodynamically
cannot
proceed.

We have seen that chemical reactions will only proceed if they are in accord with the Second Law. But it will help subsequent discussion to introduce another important concept—entropy. Understanding entropy is important because it is a key component of stability and, in fact, the Second Law can be expressed entirely in terms of entropy.

Entropy can be thought of intuitively as the degree of disorder in a system. If you throw a number of building blocks onto a surface, they are likely to fall into a disorganized pile rather than to stack up in an ordered manner. The tendency to disorder is inherent in the Second Law—ordered systems tend toward disorder, and this can be explained in statistical terms. Chemical systems respond to the drive toward disorder in exactly the same way and for exactly the same reasons as do tidy desks. Regardless of energy considerations, a chemical reaction that combines two species into one is
unfavourable
from an entropic point of view since that
increases
the order of the system (i.e. decreases its entropy), while a reaction that breaks up a single molecule into several fragments is
favoured
entropically as it
decreases
the order (increases the entropy) of the system. Accordingly, the free energy of a system incorporates within it an entropic contribution.

Catalysts are frequently involved in chemical reactions. In fact, one could confidently say that almost any chemical reaction can be
catalysed by some appropriate material. Within biological systems catalysts play a crucial role and are called enzymes. Without the appropriate enzyme(s) most biological reactions would either proceed very slowly, or not at all. Normally the product of a reaction and the catalyst for that reaction are different materials. In the above example of hydrogen and oxygen reacting to give water, the product is water and the catalyst would be some metal or metallic compound. But consider a reaction in which the product and the catalyst are one and the same, i.e., the product acts as a catalyst in its own formation. Such a reaction is termed
autocatalytic
for obvious reasons—the catalyst catalyses its
own
formation, rather than the formation of some other material. At first glance catalysis and autocatalysis may not seem too different. But a simple calculation of the rates at which the two reactions proceed reveals how spectacularly wrong that initial impression is. If one starts each of the two reactions, catalysis and autocatalysis, with just one
single
molecule of catalyst (or autocatalyst), a simple calculation reveals that the time required to make a small amount of material (say 100 grams) by each pathway is dramatically different. For the catalytic reaction the calculated time frame comes out in
billions of years.
For the autocatalytic reaction the corresponding calculated time frame works out at a tiny
fraction of a second!
A comparison of two seemingly similar processes doesn’t get more different than that. (It should be stated that the difference between the two numbers was spectacularly large because we started off in each case with just one molecule of reactant, but even with larger quantities of starting material the effect remains dramatic.) Let me jump way ahead for a moment and state that the essence of life will be found to lie in the dramatic difference between the rates of catalytic and autocatalytic
reactions. But we have quite a way to go in this discussion before the basis for that statement becomes clear.

How can that dramatic difference in reaction rate between catalysis and autocatalysis be explained? Simply put—the power of exponentials. The difference comes about because in the autocatalytic reaction, the rate of product formation proceeds
exponentially,
whereas in the catalytic reaction the rate of production proceeds
linearly,
and that difference could not be more profound. If that sounds too mathematical, let’s explain the difference by recounting the classical legend of the Chinese emperor who was saved in battle by a peasant farmer. When the emperor asked the farmer how he could reward him, the farmer took out a standard chess board and asked that he be rewarded with a quantity of rice, and that the required quantity be established by a simple formula—placing a single grain of rice on the first square, two grains on the second square, four on the third, and so on, right through to the 64th square. The request sounded absurdly modest and the emperor was surprised that the peasant would be happy with such a small reward. After all, how much rice could be needed? Half a sack, a whole sack? But the truth is that the amount of rice needed to comply with the peasant’s request is spectacularly large. Mathematically the total number of grains of rice placed on the board would be 2
⁶⁴
–1. That works out at close to 2 × 10
¹⁹
grains—that’s a lot of rice; more than could be found in the emperor’s cellars, as well as in all the world’s Chinese restaurants, and, in fact, more than exists anywhere on the entire planet. That quantity of rice, if it existed, would cover the entire earth’s surface to a depth of several centimetres.

By comparison
linear
growth, as expressed by the catalytic path, would be the equivalent of placing a
single
grain of rice on each of
the 64 squares. Hence the total amount of rice placed on the chess board would be just 64 grains! That’s 64 grains of rice (representing catalysis) compared to some 2 × 10
¹⁹
grains (representing autocatalysis). Autocatalysis is clearly an extraordinary reaction, explosive in its impact.

But do autocatalytic reactions actually exist? The answer is yes, they do, and in fact they are quite common in chemistry. For example, the reaction of acetone with bromine to give bromoacetone and hydrogen bromide is autocatalytic. That is because the reaction is catalysed by the presence of acid, and one of the products (hydrogen bromide) is an acid. Not surprisingly, the rates at which autocatalytic reactions proceed increase dramatically as the reaction progresses. However, that kind of autocatalytic reaction is not of special interest to us here. It is another kind of autocatalytic reaction, first discovered some forty years ago that is truly remarkable and enormously significant. I am referring to long chain-like molecules that are capable of making copies of themselves, molecules that are self-replicating. Sounds miraculous? It isn’t—it’s just chemistry. In 1967, Sol Spiegelman a microbiologist at the University of Illinois, performed one of the truly great classic experiments in molecular biology when he carried out molecular replication in a test tube.
²⁷

Spiegelman simply mixed an RNA strand (RNA stands for ribonucleic acid and differs slightly in structure from its more famous cousin, DNA) with free floating building blocks from which the RNA is itself built up, an enzyme catalyst to speed up the reaction, and lo and behold, the RNA strand ended up making copies of itself. Let us examine this replication reaction in greater detail. Self-replicating molecules, such as RNA, are self-replicating because
they are able to induce a supply of building blocks, from which the molecule itself is composed, to connect up, thereby making a copy of the original molecule. A schematic representation of the RNA molecule is shown in Fig. 3a and the replication process is shown in Figs. 3b and 3c. From Fig. 3a we can see that RNA is a long chain-like molecule composed of segments called nucleotides that are linked together to make up that chain. In the case of an RNA molecule there are four possible nucleotides from which the chain may be built up, which can be simply labelled as U, A, G, and C. So an RNA chain might be represented by the sequence of those four letters, e.g., UCUUGAGCC… as indicated in the figure. Accordingly, the number of possible RNA chains, each with its particular sequence of nucleotides, grows dramatically as the chain length increases. Even for a relatively short RNA chain, say 100 nucleotides in length, the potential number of different chains is staggeringly large, 4
¹⁰⁰
. That’s equal to 1.6 Χ 10
⁶⁰
—a 1 followed by 60 zeroes.