Financial Markets Operations Management (11 page)
Read Financial Markets Operations Management Online
Authors: Keith Dickinson

Whilst bonds with different maturities tend to have different yields, the conversion factors are based on pricing all the deliverable bonds at the same yield. The result of this is that one of the bonds tends to be the “cheapest to deliver” bond and this CTD bond is what tends to be delivered against a short futures position. At its simplest, a CTD bond is one that would make the most amount of money if it were purchased on credit and delivered against a short futures position.
Operationally, we do not need to know how the CTD bonds are identified. If a delivery were to take place, we would be told the EDSP and from that we could calculate the total invoicing amount. In reality, the majority of open contracts are closed before expiry and only comparatively few contracts are actually delivered.
Options have a language of their own; for example, we refer to a buyer and a seller as being a
holder
and a
writer
of an options contract respectively. We will come across other terminology as we go through the section. In the above definition we are referring to an option from the holder's point of view, i.e. the holder has the right to purchase an asset (call option) or the right to sell an asset (put option). But for every purchaser there must be a writer (seller).
It is normal practice for the holder to decide whether to exercise or not. If the holder wishes to exercise, then the writer is obliged to deliver.
For the holder, the premium is the price paid to take a view on whether it would be advantageous to exercise the contract at the appropriate time. For the writer, the premium is the price received as partial compensation if they are obliged to exercise the contract. We refer to options as
premium settled
.
More terminology: the price of an options contract is known as the
premium
.
Here we have an example of a nonlinear price relationship. If we look at a break-even chart, we will observe that the profit or loss line is broken at the point denoted by the premium amount.
The following four figures illustrate the pay-offs for the call and put options (all with a strike price of 100 and a premium of 10):
- Long call option (see
Figure 2.4
); - Short call option (see
Figure 2.5
); - Long put option (see
Figure 2.6
); - Short put option (see
Figure 2.7
).
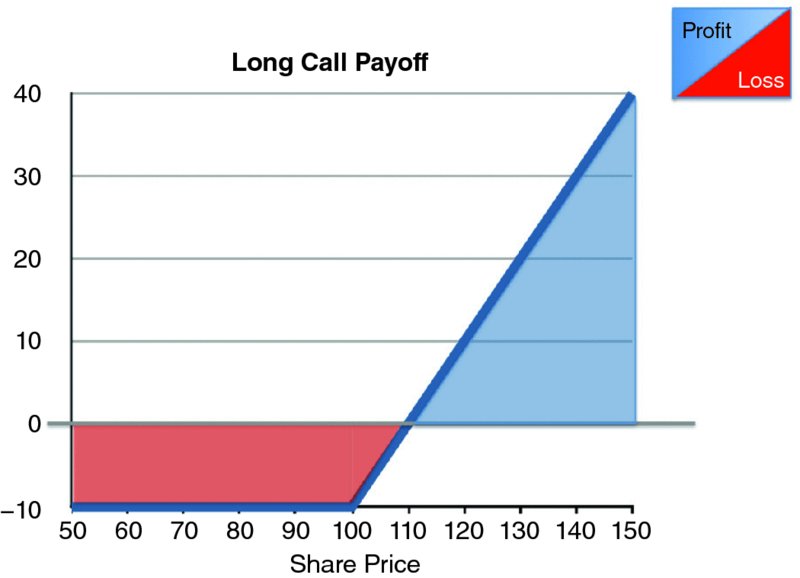
FIGURE 2.4
Long call option
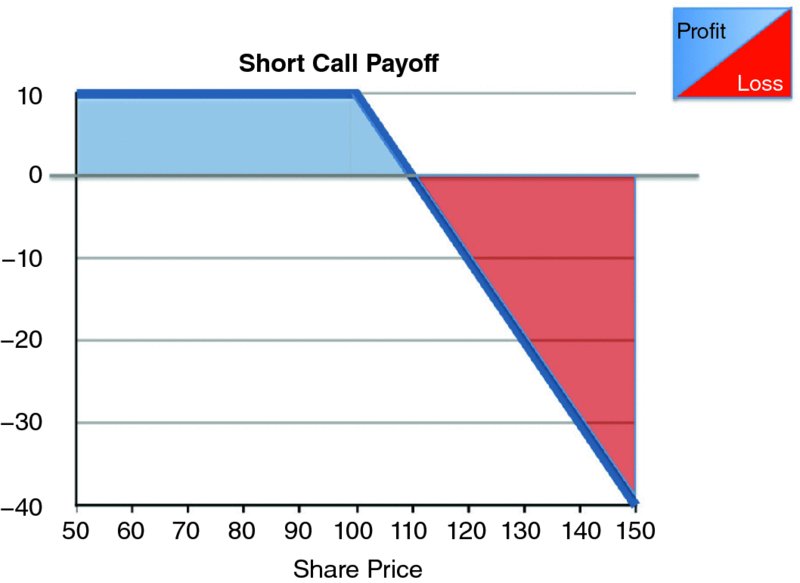
FIGURE 2.5
Short call option

FIGURE 2.6
Long put option

FIGURE 2.7
Short put option
The value of a call option rises as the underlying market price rises. If you buy a call option, you make money as the underlying market price rises. If the market price falls, your loss is limited to the premium you paid.
If you sell a call option, you lose money as the underlying market price rises. If the market price falls, your profit is limited to the premium you received. Not a particularly attractive
risk/reward profile â but not dissimilar to the insurance business. The level of premium/price received is key.
The value of a put option rises as the underlying market price falls. If you buy a put option, you make money as the underlying market price falls. If the market price rises, your loss is limited to the premium you paid.
The value of a put option rises as the underlying market price falls. If the market price rises, your profit is limited to the premium you received.
In the same way that futures contracts are specified by a derivatives exchange, so, too, are options contracts. Options contracts will usually contain the specifications outlined in
Table 2.44
.
TABLE 2.44
Options contract specifications
| Contract Specification | Description |
| Option type | Call option (holder has the right to buy) Put option (holder has the right to sell) |
| Option style | American style â the option can be exercised at any time up to expiry of the option European style â the option can only be exercised on one date in the exercise month |
| Quantity and class of the underlying asset | For example, one contract relates to 1,000 ABC ordinary shares |
| Strike price (or exercise price) | The price at which the underlying transaction will be exercised |
| Minimum tick size | The minimum price movement |
| Contract month | Usually based on a quarterly cycle |
| Expiry date | This is the last date on which the contract can be exercised |
| Settlement terms | Whether settlement is by the actual delivery of the underlying asset (against cash) or by an equivalent amount of cash only |
For an example of the underlying shares for an options contract including Canon Inc., see
Table 2.45
.
TABLE 2.45
Canon Inc. option contract specifications
| Contract Specification | Description |
| Option type | Call and put options based on listed securities selected by the Tokyo Stock Exchange |
| Option style | European style |
| Quantity and class of the underlying asset | 100 Canon Inc. shares Local code: 7751 ISIN code: JP3242800005 |
| Strike price (or exercise price) | Exercise prices with the intervals based on the last execution price of the underlying security set on the first trading day |
| Minimum tick size | Depends on the size of the option premium, e.g. if the premium is between JPY 1,000 and JPY 3,000 per share, then the tick size is JPY 1 |
| Contract month | Two closest serial months plus two closest quarterly months (March, June, September, December) |
| Expiry date | The last trading day (i.e. European-style option) |
| Last trading date | The business day prior to the second Friday of the respective contract month |
| Settlement terms | Cash settlement and physical delivery of the underlying on the fifth day counting from the day of exercise |
Source:
Tokyo Stock Exchange (online). Available from
www.tse.or.jp/english/rules/derivatives/eqopt ions/index.html
. [Accessed Sunday, 15Â December 2013]
Before we look at the pricing of options, we need to introduce some further terminology.
There is a relationship between the price of an underlying asset and the strike price of an option. The ITM and OTM positions in
Table 2.46
refer to the holder of the option. It will be the opposite for writers.
TABLE 2.46
ITM/OTM/ATM for option holders
| In-the-money | ITM |
|
| At-the-money | ATM |
|
| Out-of-the-money | OTM |
|
We can now summarise the risks associated with futures and options contracts (see
Figure 2.8
).
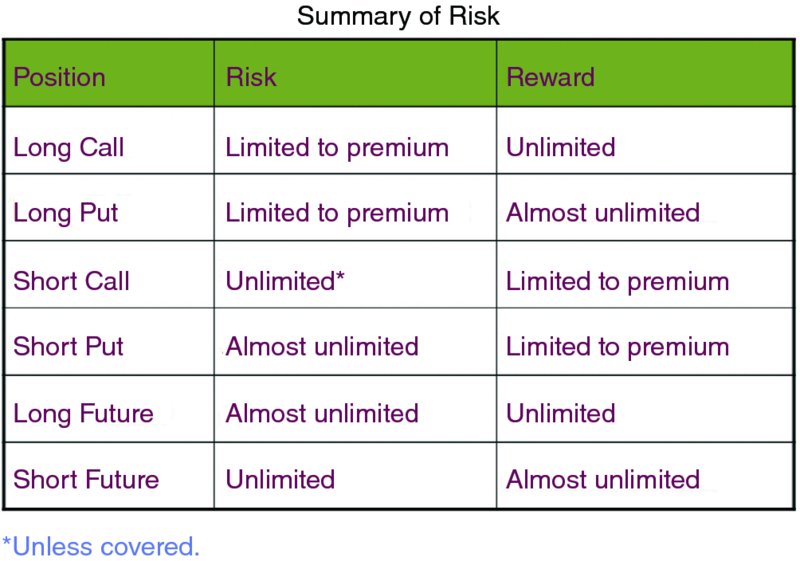
FIGURE 2.8
Comparison of the risks of futures and options