To Explain the World: The Discovery of Modern Science (47 page)
Read To Explain the World: The Discovery of Modern Science Online
Authors: Steven Weinberg

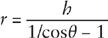
For instance, on a mountain in India al-Biruni found
θ
= 34’, for which cos
θ
= 0.999951092 and 1/cos
θ
– 1 = 0.0000489. Hence
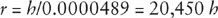
Al-Biruni reported that the height of this mountain is 652.055 cubits (a precision much greater than he could possibly have achieved), which then actually gives
r
= 13.3 million cubits, while his reported result was 12.8 million cubits. I don’t know the source of al-Biruni’s error.
17. Geometric Proof of the Mean Speed Theorem
Suppose we make a graph of speed versus time during uniform acceleration, with speed on the vertical axis and time on the horizontal axis. The graph will be a straight line, rising from zero speed at zero time to the final speed at the final time. In each tiny interval of time, the distance traveled is the product of the speed at that time (this speed changes by a negligible amount during that interval if the interval is short enough) times the time
interval. That is, the distance traveled is equal to the area of a thin rectangle, whose height is the height of the graph at that time and whose width is the tiny time interval. (See Figure 11a.) We can fill up the area under the graph, from the initial to the final time, by such thin rectangles, and the total distance traveled will then be the total area of all these rectangles—that is, the area under the graph. (See Figure 11b.)

Figure 11. Geometric proof of the mean speed theorem.
The slanted line is the graph of speed versus time for a body uniformly accelerated from rest. (a) The width of the small rectangle is a short time interval; its area is close to the distance traveled in that interval. (b) Time during a period of uniform acceleration, broken into short intervals; as the number of rectangles is increased the sum of the areas of the rectangles becomes arbitrarily close to the area under the slanted line. (c) The area under the slanted line is half the product of the elapsed time and the final speed.
Of course, however thin we make the rectangles, it is only an approximation to say that the area under the graph equals the total area of the rectangles. But we can make the rectangles as thin as we like, and in this way make the approximation as good as we like. By imagining the limit of an infinite number of infinitely thin rectangles, we can conclude that the distance traveled equals the area under the graph of speed versus time.
So far, this argument would be unchanged if the acceleration was not uniform, in which case the graph would not be a straight line. In fact, we have just deduced a fundamental principle of integral calculus: that if we make a graph of the rate of change of any quantity versus time, then the change in this quantity in any time interval is the area under the curve. But for a uniformly increasing rate of change, as in uniform acceleration, this area is given by a simple geometric theorem.
The theorem says that the area of a right triangle is half the product of the two sides adjacent to the right angle—that is, the two sides other than the hypotenuse. This follows immediately from the fact that we can put two of these triangles together to form a rectangle, whose area is the product of its two sides. (See Figure 11c.) In our case, the two sides adjacent to the right angle are the final speed and the total time elapsed. The distance traveled is the area of a right triangle with those dimensions, or half the product of the final speed and the total time elapsed. But since the speed is increasing from zero at a constant rate, its mean value is half its final value, so the distance traveled is the mean speed multiplied by the time elapsed. This is the mean speed theorem.
18. Ellipses
An ellipse is a certain kind of closed curve on a flat surface. There are at least three different ways of giving a precise description of this curve.
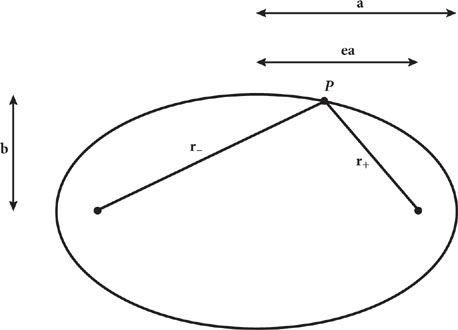
Figure 12. The elements of an ellipse.
The marked points within the ellipse are its two foci;
a
and
b
are half the longer and shorter axes of the ellipse; and the distance from each focus to the center of the ellipse is
ea
. The sum of the lengths
r
+
and
r
–
of the two lines from the foci to a point
P
equals 2
a
wherever
P
is on the ellipse. The ellipse shown here has ellipticity e 0.8.
0.8.
First Definition
An ellipse is the set of points in a plane satisfying the equation

where
x
is the distance from the center of the ellipse of any point on the ellipse along one axis,
y
is the distance of the same point
from the center along a perpendicular axis, and
a
and
b
are positive numbers that characterize the size and shape of the ellipse, conventionally defined so that
a
≥
b.
For clarity of description it is convenient to think of the
x
-axis as horizontal and the
y
-axis as vertical, though of course they can lie along any two perpendicular directions. It follows from Eq. (1) that the distance of any point on the ellipse from the center at
of any point on the ellipse from the center at
x
= 0,
y
= 0 satisfies
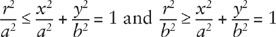
so everywhere on the ellipse
